TEXT: Intermediate Algebra by Miller, O’Neill & Hyde
Videos from Khan Academy.
To solve a radical equation, by which we mean an equation that has one or more radical expressions with variables in the radicand, our textbook provides a procedure:
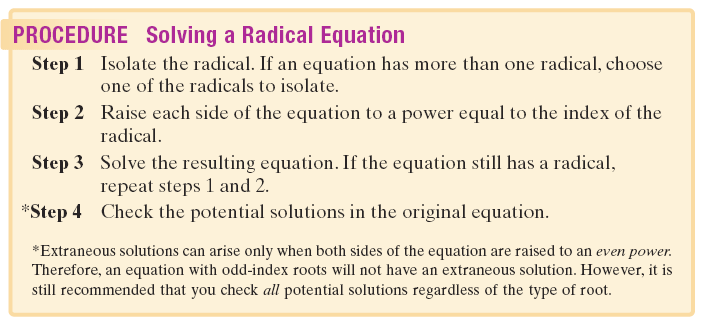 Much of the procedure is geared for a more general situation than we will see. Here is a procedure more tailored to your homework and exam questions:
Much of the procedure is geared for a more general situation than we will see. Here is a procedure more tailored to your homework and exam questions:
PROCEDURE Solving a Radical Equation
Step 1 Isolate the radical expression (or one if them if there are multiple radicals).
Step 2 Square both sides of the equation.
Step 3 Once the radical expressions have been eliminated, solve the resulting equation.
Step 4 Check the potential solutions in the original equation.
Some notes:
- Sometimes there are multiple radicals. For your exercises, all the radicals will be eliminated with step 2. If not, repeat steps 1 and 2.
- Checking each solution into the original equation is a necessary part of the solution process. Squaring both sides of an equation can introduce extraneous or false solutions.
Here are 3 progressively harder but relatively easy examples:
Here is a much more difficult problem which demonstrates the issue of extraneous solutions mentioned earlier [I highly recommend that you watch this!]:
The following application from physics involves the kinetic (moving) energy Ek of a bowling ball traveling at a velocity (speed) v:
Alternatively, we could have solved for the kinetic energy Ek first and then substituted the given values. This is especially useful if you want to calculate the energy for several different values of the other variable(s).




This lesson is little harder then other because, we have to solve the problem with longer steps.. Sometimes we have to factor or sometimes we have to find the GCF of the equation.