TEXT: Intermediate Algebra by Miller, O’Neill & Hyde
Videos from Khan Academy.
A rational expression is a ratio of polynomials (p/q) with nonzero denominator (q≠0).
A rational expression can be simplified using the following principle:
 It is a good idea to always put a property or procedure into your own words. Do that now before proceeding.
It is a good idea to always put a property or procedure into your own words. Do that now before proceeding.
Here is the first video:
The next 2 videos do more complicated situations and also find the domain. Rational expressions can be thought of as functions. The domain of a function is where it is defined (technically the set of inputs). To find the domain of a rational function, you find where the denominator is 0 and then exclude those points.
Multiplication of rational expressions relies on:
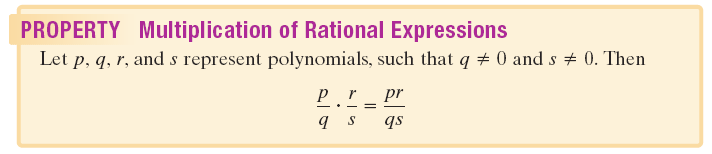 The first video does an example where the polynomials in our rational expressions are monomials:
The first video does an example where the polynomials in our rational expressions are monomials:
The next video gives a more complicated example:
To divide, we use the following principle to transform the quotient into a multiplication of rational expressions (and then use the multiplication property):
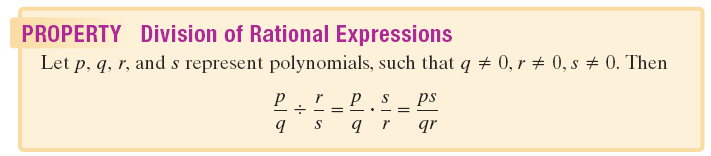 Put the property (or procedure) into your own words before proceeding.
Put the property (or procedure) into your own words before proceeding.
Here is a video presenting an example:




I figure out how to this from mathzone
I am little confused about this lesson, I did not get to we can state the domain.
I need more help with stating the domain.
The tricky thing is the logic. By setting the denominator to 0 and solving for the variable, you are not finding the domain. You are finding what is known as the complement of the domain. Visually, the domain for a rational function is going to be the entire real number line with some holes in it. Those holes are precisely where the denominator is 0.
I actually had fun in class its becoming more interesting
Pro. Halleck
Okay so I get it, but would have like to do more division examples