Question and Solution for Test #1 Review
Month: February 2020 (Page 2 of 4)
I’ve received a few questions about what to expect on Test #1. Here are some answers.
- There will be 10-15 questions total.
- Questions will be graded just like quiz questions: total of 10 points per question including 1 point for style and 3 for checking antiderivatives by differentiating (where appropriate): shorter questions will be worth 5 points each.
- No formula sheets are allowed. You must memorize the relevant trig identities as well as derivatives and antiderivatives.
- You must show all your work. If no work is shown but an answer is given without justification, a report will be filed with the Academic Integrity Committee.
- You may use a calculator, but you may not use any other devices (you may not use your phone’s calculator).
- You will have the whole class period but time will be an issue. Make sure you complete your practice problems several times so that you can complete them quickly.
- When you are applying a particular integration technique, state which one it is you are applying.
This week I’ll be in my office (probably!) around 11:30 or 12:00 noon; check in at N707.
Tomorrow (Thursday) I’ll be in my office N707 from 12:00 noon to about 12:30.
Test #1
Test #1 will be given in class on Monday, March 2 and will cover material/homework from Sessions 1-7 (in pink on the schedule). The test will take the full class period and will include 10-15 questions similar to questions from the textbook, Webwork, and the final exam review sheet.
Test #1 Review Assignment – short version
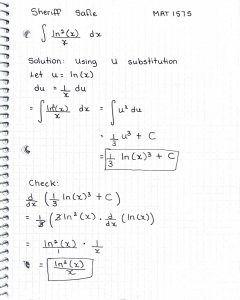
To prepare for the test, you as a class will construct a crowd-sourced review sheet. Each of you is responsible for choosing one question and posting the question and its solution on the OpenLab. Try to pick questions that you think make good test questions (not too easy); try to pick topics that haven’t been posted by your classmates. Select the category Test #1 Review from the right-side of the screen before posting your question/solution. Your post is due on the OpenLab by Friday, February 28 at 11:59pm.
Test #1 Review Assignment – detailed instructions
Choose one question from
- the textbook homework on the course outline,
- Webwork, or
- the final exam review sheet
to complete and post your full solution on the OpenLab. Title your post “Test #1 Review” and add the section and problem number. Add the category Test #1 Review before submitting your post. You may type out the solution or upload a photo of hand-written work.
Make sure nobody has submitted your problem already; try to make sure every section that will be on Test #1 is represented.
It is up to you as a class to ensure that all solutions are correct. If you have a question about someone else’s post, if you would like more detail, or if you think the solution contains an error, post a comment asking the question or correcting the error.
The idea here is that you as a class are creating a review sheet for everyone to study from for the first test. You will be given participation credit for this post.
Due date: Friday, February 28 at 11:59pm
I wanted to return to the example we were considering in class today, before too much time passes and we forget where we were.
Recall, we were trying to evaluate the integral
We saw that if
This means that
After simplifying, this is equal to
This is how far we got in class. The point is that this is an integral we know how to evaluate.
To continue,
To finish, we have to go back to our dictionary to write our answer in terms of
To replace the
To replace
This means our final answer is:
$\int \frac{\sqrt{x^2-9}}{x}dx = 3(\tan(\theta) – \theta) + C = 3(\frac{\sqrt{x^2-3}}{3} – \sec^{-1}(\frac{x}{3})) + C
= \sqrt{x^2-3} – 3\sec^{-1}(\frac{x}{3}) + C$.
A few people asked during class, “Where does
In general, to make a trigonometric substitution:
- if you see
- if you see
- if you see
This should be enough for you to get a solid start on the Trigonometric Substitution Webwork set, which is due on March 1.
Webwork
- Integration by Parts due tomorrow
- Trigonometric Integrals due Sunday
- Trigonometric Substitution due March 1
Class Schedule
- No class this Wednesday
- No class next Monday
Quiz #2
- Quiz #2 will be given in class at the beginning of class on Wednesday, February 19. It will cover material from Sessions 3, 4, and 5.
Trigonometric Substitution
- After next Wednesday’s quiz, we’ll start the next topic: trigonometric substitution. You’ll want to make sure that you remember your trig identities and derivatives to prepare for this topic.
Test #1
- Trigonometric substitution is the last topic that will appear on Test #1, which will be given in class on Monday, March 3. This might sound far away, but you’ll want to start preparing now. You won’t be told which integration technique to use, like you are in Webwork; you’ll have to decide on the best technique yourself (there may be more than one appropriate technique to choose for a given problem). Make sure to practice exercises where you are not told which technique to use. See the final exam review sheet and the end-of-chapter review exercises in the textbook.
Test #1 Review – OpenLab Assignment
Stay tuned for your next OpenLab assignment (spoiler: you’ll choose and solve one review question for Test #1 and post it to the OpenLab… details TBA).
I mentioned during office hours today that I work closely with the director of the Atrium Learning Center and with the math tutors who work there. One of the things I do each semester is create a flyer to encourage students to attend tutoring. For the past few semesters, I’ve used this meme combo:
These memes are getting a little old, so I was thinking of changing them up this semester. I’ve come up with a few ideas of my own, but I thought it’d be fun to ask you to come up with your own too. This assignment is optional and purely for fun but anyone who creates their own meme will earn one participation point (you can enter as many times as you want, but you’ll still get only one point for entering). If one of your memes is ultimately chosen for the official poster, you’ll be declared the winner and will earn two extra participation points. Continue reading
- A new Webwork feature has just been enabled. When you are logged in and looking at one of your questions, you’ll see a button at the bottom labelled “Ask for Help.” Clicking on this button will take you to an OpenLab page which is a question-answer forum for that question specifically (each student has the same template for each question; the numbers may be different but the procedures for answering the questions will be almost the same). If there’s a Webwork question that’s troubling you, please submit the details on the “Ask for Help” page and/or scroll down to check if your question has been submitted previously by someone else. You’ll get participation credit for asking your own question or answering someone else’s on this page.
- Tutoring is available in different places around campus. The schedule for the Atrium Learning Center is available here: PERKINS MATH TUTOR SCHEDULE FOR SPRING 2020. You’ll get participation credit for attending tutoring; ask the tutor for a verification slip and give it to me.
The Webwork set “Substitution Method” is due next Sunday night. Many of the exercises are similar to the example we saw in class today. We’ll see another example or two after Thursday’s quiz, before we start the next section: Integration by Parts.






Recent Comments