Announcements
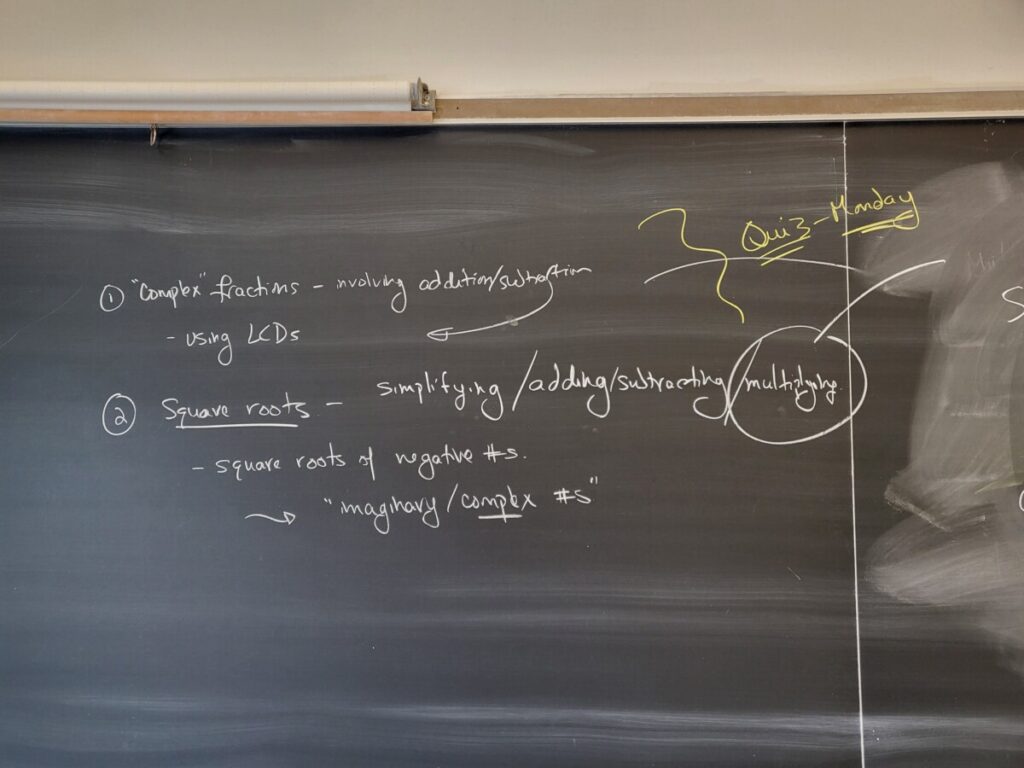
We will have a quiz on Monday , covering the basics of simplifying and adding/subtracting radicals (square roots), as well as complex fractions.
I have reopened/extended some of the WebWork sets so you can work on these if you didn’t complete them earlier:
- Radical Expressions-Adding and Subtracting – due Sun March 24 (Sec 1.4.4)
- Rational Expressions-Complex Fractions 2 – due Mon March 25 (Sec 1.3.4)
- Radical Expressions-Multiplying – due Wed March 27 (Sec 1.4.4)
- Radical Expressions-Complex Numbers – due Fri March 29 (Sec 1.4.6)
Topics
We did a couple more examples of simplifying complex fractions, using the “keep-change-flip” technique:

We then did a complex fractions example (from the “Complex Fractions 2” WebWork) where we first have to do addition/subtraction using least common denominators (to get it into a form where we can “keep-change-flip”):

We did an example from the WebWork of multiplying square roots epxresions:
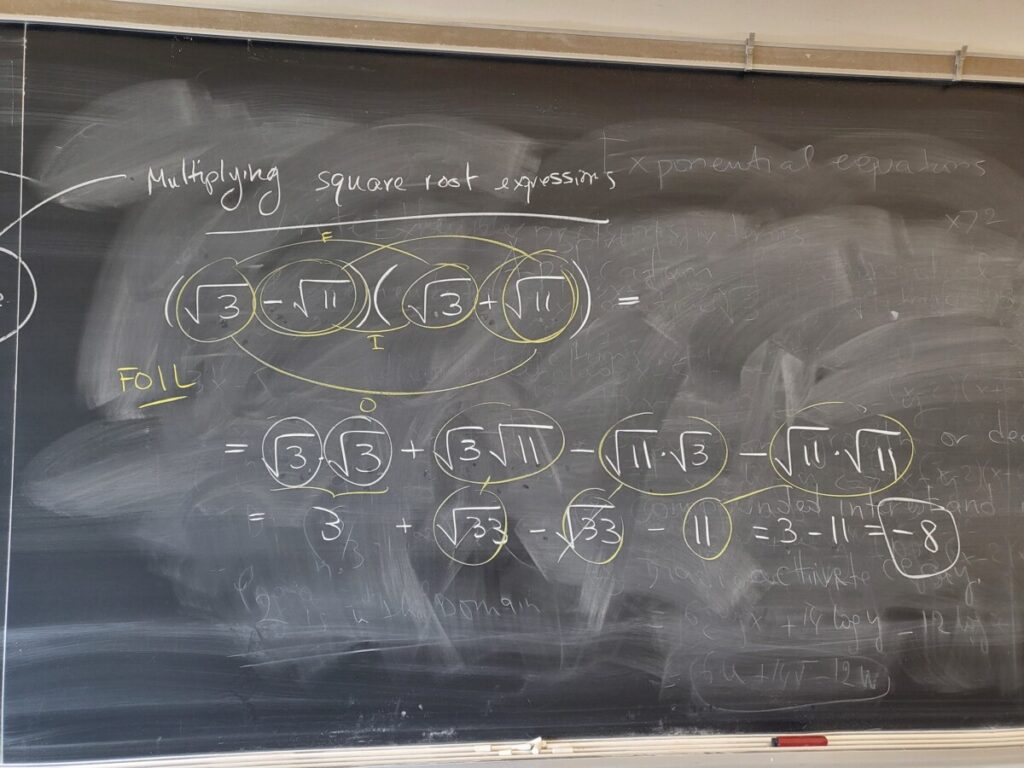
We then introduced “imaginary number” symbol i, to represent the square root of -1, and showed how we can use this to represent the square root of a negative number, such as in the solutions of a quadratic equation from the quadratic formula:

We introduced operations on these “complex numbers” by verifying that checking that one of the results we got from the quadratic formula is actually a solution of the quadratic equation:

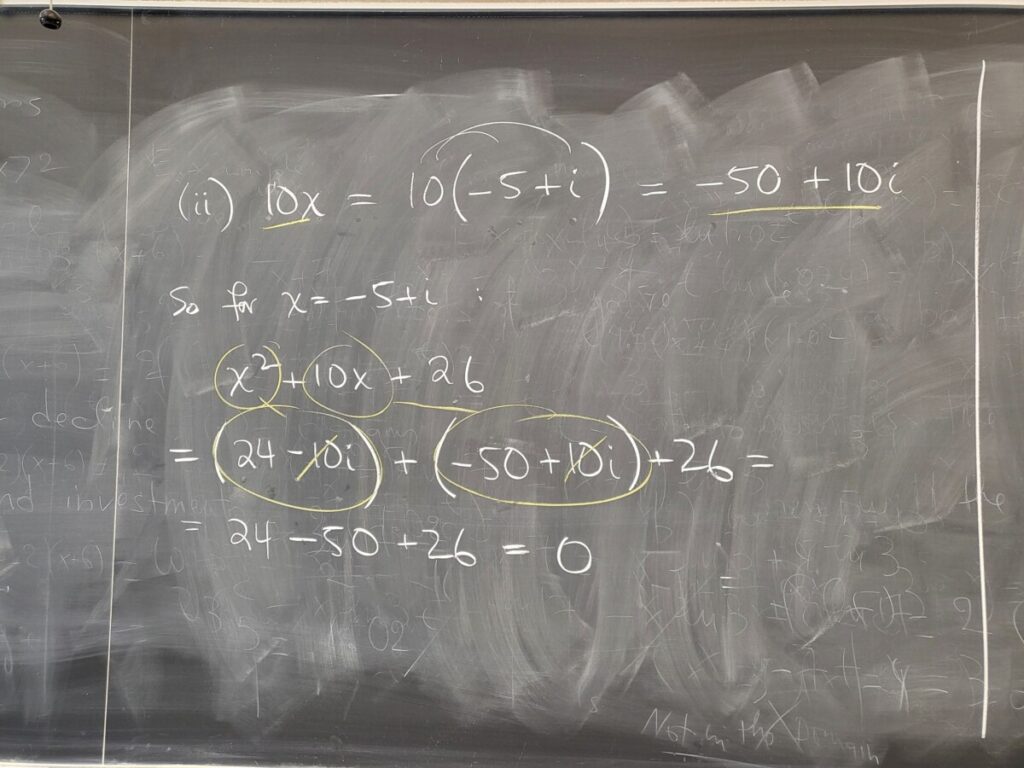
We went back and defined such complex numbers as expressions of the form “a+bi” (where a, b are real numbers), how to do addition and multiplication on complex numbers:
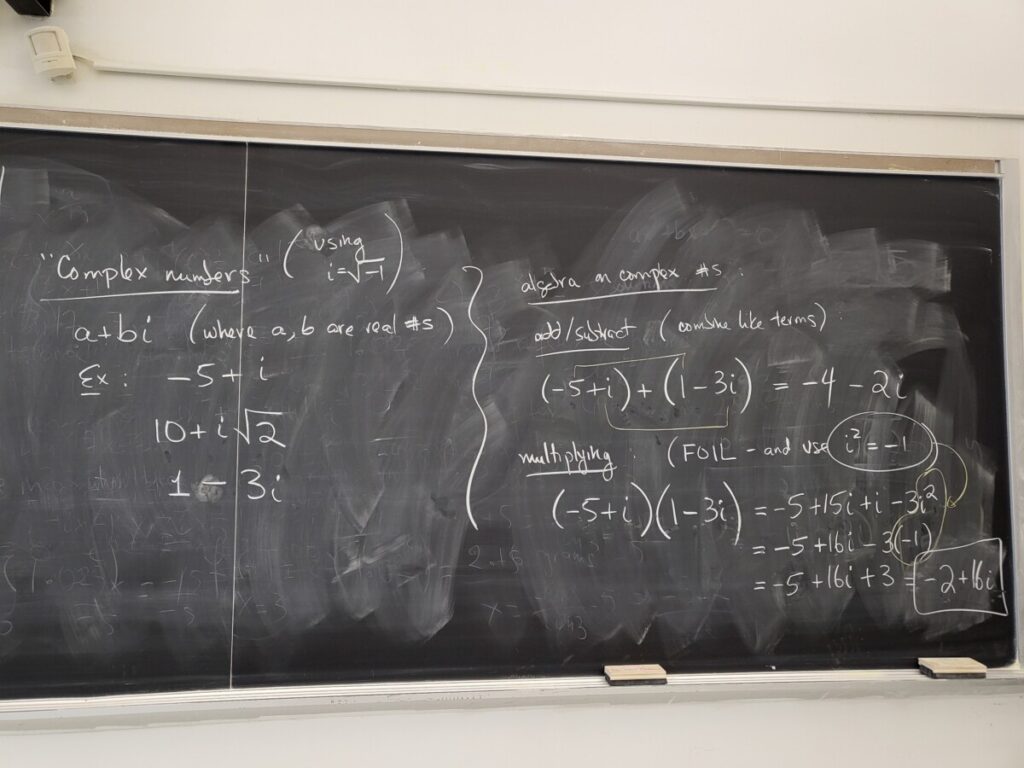



Recent Comments