MAT1575 Calculus II Spring 2015

Here’s a pretty good interactive applet for visualizing Riemann sums for different functions, different intervals, different numbers of rectangles, and different points in subintervals used to calculate heights of rectangles. The idea is that you’ll fix f(x), a, b, and p, and let the number of rectangles (n) increase.

Here are a few interesting charts generated from Calculus II scores.

The first is the grade distribution for test #1. There are two noticeable peaks; a number of students earned grades in the 30’s and a number of students earned grades in the 60’s/70’s.

The second compares test #1 grades with WebWork grades. There seems to be a pretty obvious positive correlation between WebWork grades and test #1 grades, with WebWork grades as slightly higher than test #1 grades.

The third uses data from my MAT 1575 class from last semester. Last semester the test that covered sequences and series was test #3, toward the end of the semester. In particular, that test was taken only by students who remained enrolled in the class after the drop date. As you can see, there is a correlation between those students’ test #3 grades and their grades on the final exam. In particular, students’ test #3 grades were pretty good predictors of their final exam grades.

Today’s the day that some people are calling the Pi Day of the Century. You’ve probably heard that is an irrational number, which means its decimal expansion never ends and never repeats. Some of you saw a really cool talk in the Math Club on Thursday that showed why. The decimal expansion begins 3.14159265359….Today’s date (in MM/DD/YY format) is 3/14/15, so day is celebrated today at 9:26:53. The traditional way to celebrate is to eat pie…too much pie.

The constant arises in many ways in math and in nature (not just from circles). Since we just finished our section on infinite series, I thought I’d mention one here. Since we know that , we also know that . Therefore, is equal to . Now, if you were asked to find the Taylor series for the function centered at , you’d eventually answer T(x)= x–​​1/3x^​3+​1/5​​​​x^​5​​​-1/7x^7+…. This means that the Taylor series for centered at is 4T(x) =4(x−​1/3​​x^​3​​​​+​1/5​​x^​5​​​​−1/7x^7+…​). Since converges to , converges to , which is . But 4T(1) = 4(1-1/3+1/5​​​-1/7+…). So here we have an infinite series that converges (which test would you use?) and it converges to .

Here are some links about and about day.

Why Pi Matters by Steven Strogatz

Mathematics Much More than Pi Day by Darren Glass

Happy Pi Day 2015 by Hojae Lee

Neil deGrasse Tyson’s Twitter…containing a fun tweet storm about day (among other nerdy things)

Clip from Star Trek Spock knows what’s up: isn’t just irrational, it’s also transcendental. This means that can’t be the solution to any polynomial equation.

A few people have asked about the limit comparison test during office hours. It’s stated on page 564 of your text, but we can reword it in a way that might make it easier to understand.

First, imagine that instead of assuming that 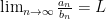, we assumed that  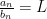 for all values of n, not just in the limit.

If 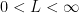, we could write 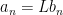and . Either way, 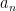is a multiple of 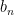and vice versa. Multiplying a series by a constant (non-zero, non-infinite) doesn’t change whether it converges or diverges. Therefore, either both series and 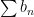converge or they both diverge….both series have to behave the same way.

If 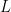is very very large, then 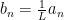, that is, 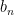is a tiny multiple of 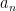. Therefore, if you know that converges, then 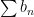must also converge.

If 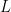is very very small, then 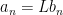, that is, 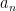is a tiny multiple of 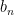. Therefore, if you know that 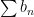converges, then must also converge.

None of what I’ve said so far should sound too crazy. What’s interesting about the limit comparison test is that you don’t have to assume that 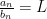for all values of , you just have to assume that 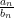 is close to 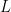only for large values of . If 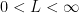, what this says is that 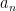is eventually almost a multiple of 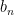and vice versa. Therefore, either both series and 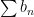converge or they both diverge…both series have to behave the same way.

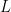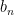Additionally, if you let actually approach , then  is eventually almost a tiny multiple of 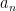. Therefore, if you know that converges, then 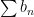must also converge.

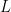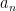Finally, if you let actually approach , then is eventually almost a tiny multiple of 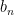. Therefore, if you know that 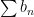converges, then must also converge.

tl;dr: If the individual terms of one series are eventually almost equal to a multiple of the individual terms of another series, then both series have to behave the same way (both converge or both diverge).

(1) WebWork Power Series (10.6) and Taylor Series (10.7) are due Monday night.

(2) Your test on Tuesday will cover everything we’ve seen so far: sections 8.4, 10.1-10.7 (skip the integral test). Be prepared to answer true/false questions and to state definitions and theorems precisely.