(after Test 3)
Topics:
• The inverse trig functions
• Solving right triangles using the inverse trig functions
Definitions and how to read the notation:
Note: the superscript -1 in these functions’ names represents the inverse function. It is very important that you remember that this is not the -1 power.
An infinite number of angles (arcs on the unit circle) can have the same sine x. The inverse sine picks out the one angle which is between
Definition:
Note that the sine of an angle must be between -1 and 1 (inclusive), therefore
similarly:
An infinite number of angles (arcs on the unit circle) can have the same cosine x. The inverse cosine picks out the one angle which is between
Definition:
Note that the cosine of an angle must be between -1 and 1 (inclusive), therefore
An infinite number of angles (arcs on the unit circle) can have the same tangent x. The inverse tangent picks out the one angle which is between
Definition:
Homework:
• Review the example we discussed in class. (My WeBWorK problem)
• Do the WeBWorK assignments. It is recommended that you do them in the following order:
(1) Unit Circle (from last time)
(2) Graphing Sine Cosine (also from last time)
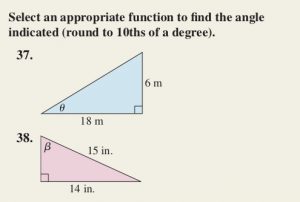
(3) Solving Right Triangles – Inverse Trig
• Here are problems (including the promised problems from Coordinate Plane Definition) from the textbook which you may put on the board at the start of class next time:
On the coordinate plane definition of the trig functions:
Find the values of the six trig functions of
(1) (-6, 8)
(2) (5, -12)
(3) (-7, -7)
From the Trig textbook:
Remember that if you get stuck on any of the problems or have a question about any of the material, you can post a question to the Piazza discussion board.
In case you prefer to view videos on any of these topics, Here is a copy of the Course Outline with links to video resources for each topic.