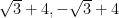OpenLab Assignment (Due next Tuesday evening, March 16th). Your second exam will take place next week. The review sheet has been posted. In the interest of making this assignment a help (instead of an extra chore), I’m asking you to post a comment related to the second exam. Any one of the following types of comments will earn you full points:
- A request for help on a question. It must be more specific than just “how do I do this problem?” Let us know where you’re stuck (and what you’ve done so far). If you don’t know how to get started on a problem, you can say so — but tell us a little about why (is it different from problems we did in class?). Your post must use correct
notation!
- A general question about a topic that we’ve been studying. Is there some part of the course that’s been bothering you? A type of problem that you just don’t get? Something you keep trying but always get wrong? Post a question here – maybe someone can help.
- An answer to someone else’s question. You don’t have to give every step or every detail, but you should provide enough information to help them along. Your post must use correct
- A helpful comment or suggestion about a topic that we’ve been studying. Do you have a trick for solving certain problems? A neat way of remembering a formula? Some thoughts about what makes certain problems hard, and suggestions for solving them? Post it!
WeBWorK Update: As you all know, WeBWorK access has been terrible this week! Our hardworking system administrator is trying to figure out what’s going wrong, but until the difficulties get worked out I am making the following changes:
- WeBWorK assignment #1 will be extended for 2 weeks, and is now due on March 20th (this update will be made on the WeBWorK servers as soon as I can get access to them…).
- There will be no new WeBWork assigned until the current difficulties are resolved. We’ll pick up again once the system is working more reliably.
- Some of you may have noticed Assignment #2 in the WeBWorK system. This will be removed shortly — you don’t have to worry about it.
I hope this addresses your concerns about our current WeBWorK problems!
The toughest topic for me that will most likely show up on exam 2 is solving a quadratic equation using the method of completing the square with fractions. The method of completing the square gets way too difficult when fractions are involved because there are quite a few things to remember concerning fractions in addition to remembering how to do the problem itself. Is there an easy way to remember what to or what not to do when fractions show up in completing the square?
-Andrew Boodhoo
There have been a few comments about completing a square with fractions — and you’re right, it’s tricky! I’m going to put a link to a video (from Khan Academy — they are awesome) in which he goes through one of these step by step, messy fractions and all. If you’re having trouble, watch this and try to work through it with him:
NOTE: the video should start partway through (at about 8 minutes) — If you rewind to the beginning, he does another example of completing the square, without all the fractions (but with a fair bit of explanation).
Hope this helps, at least a little!
Mr. Reitz
The hard part for me is when im doing the homework i have my notes and the examples from the textbook..but when the exam comes i never can remember how each method goes ive gotten so used to solving all quadratic equations using the formula that any other way is so confusing… and the other problem im having is how far to simplify the answer there are so many rules for different things it just becomes stressful especially during the test :/
The tough part for me is remebering every step and different methods to solve a problem.Especially when it comes down to the test day I struggle which method I have to use to solve that specific problem especially if it involves fractions since im so weak in those. – Edwin Zapata
I think completing the square will be (is) the one that I’m most stumped on. That middle step is a killer, especially if it gives a fraction as an answer. Trying to remember steps 1 – whatever is hard by itself, but when a fraction get thrown in the mix its like “hold up…what???” so i def see myself being stuck on that. But I get the quadratic formula and the others.
-Demetria Anderson
Oh and I have a problem from the review sheet that has me puzzled. Its a quadratic: 5x(x-1)=x-5
After i distributed the 5x and brought the x-5 over to the other side i got 5x^2-6x +5=0
So did I do something wrong?
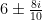
This was my answer however on the answer key the answer is: \frac{3}{5}\pm\frac{4}{5}i
Okya, that copied that wrong. the answer i got was:
latex \frac{3}{5}\pm\frac{4}{5}i$
Hi Demetria,
.
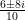You’re very close! Just be careful when you first plug into the quadratic formula — the fraction extends across the whole thing, so denominator (in this case 10) should be underneath the 6 as well as underneath the radical. In this end, this gives:
Next factor a 2 out of the top, and then cancel with the 10 on the bottom. To get the final answer, split the fraction up into two separate fractions withe same denominator.
Hope this helps – write back if you’re still stuck.
Mr. Reitz
okay i got it now. thanks 🙂
$latex\frac{3}{5}\pm\frac{4}{5}i$
I think all you need is a space after the word “latex” 🙂
-Mr. Reitz
idk y it’s not showing but its number 9b on the answer sheet 3/5 (plus or minus) 4/5i was the answer given
I think problem in class you can do, then when it comes to review sheet and test it is something else. these problem are tricky, on this sheet and i hate fractions!
sqrt9x$
There was this prob review sheet probably simply but i am stuck, 2) b. 5x
now take a 3 out of the 18 and the 15, which will now give you:
Hope this helped =)
Thanks it did, I see what i did wrong now. I messed up the multiplication of denominator some how, i had got 162. Thanks a lot 🙂
2) b. 5xsqrt3/2x^2sqrt9x
2) b.
That is prob but I can’t get right latex thing 2) b. 5xsqrt3/2x^2sqrt9x
It was hard for me to remember the quadratic formula and I found a way to remember the formula by sayaing “One sad boy
(-b)couldn’t decide (+-)to go into a radical party(√)where he saw one boy squaring off(b²) and four awesome chicks leaving the party(-4ac)The whole thing was over at 2AM (/2a)” or if that doesn’t help, make a tune in your head and sing these lyrics,
x is equal to negative b
plus or minus the square root
of b squared minus 4ac
ALL over 2a
Hope it helps :] -Talia Ordonez
Sweet – I love this!
Thanks, i love this song. Helped me remember the equation.
Reviwe sheet Exam
question #3 d) x-3√x-5=5
For the problem 3x^1/3, how or what do are you suppose to the with the exponent..seems like a silly question ><"
$latex\3x^1/3$
Okay I am going to tell a story about stuffed animals and I want to see if you can remember the Quadratic formula.
b= bear
a= apes
c= cats
At seven my mother wanted to take my bear= -b,
away because she didn’t know weather it would be good or bad for me growing up=
\[\pm \sqrt \]
I tried to get 2 more bears = \[b^2\]
but she said No take 4 apes and a cat instead = -4ac
I never wanted a cat, and I never got got over the 2 apes she brought for me anyway = \[/2a\]
Now try putting it together and tell me if it is easier to remember the formula.
Nice! These stories are a great mnemonic device.
What most likely would be on exam 2 and is tough for me is remembering how to use the complete the square method. I always, for some reason, forget to get the x^2 by itself. I usually just keep going and get a weird answer at the end, and realize i forgot to make the quadratic equation x^2.
Ex from review sheet:
Completing the square:
b) 2x^2=6x+10?/ i always forget to divide the number in front of x^2 in order to get x^2 by itself.
i think completing the squares is the most difficult in the quadratic equation. I mean, i understand the concept of it, but doing it is the problem. I have the most problems when it comes to the fractions, I’m more comfortable with whole numbers. I always seem to forget that I have to divide b by 2, square it, and then add it on. How do I make sure that I remember this step?
Here is the;
Steps for Completing the Square:
1. Be sure that the coefficient of the highest power is one.
If it is not, divide each term by that value to create a leading coefficient of one.
2. Move the constant term to the right hand side.
3. Prepare to add the needed value to create the perfect square trinomial. Be sure to balance the equation. The boxes may help you remember to balance.
4. To find the needed value for the perfect square trinomial, take half of the coefficient of the middle term (x-term), square it, and add that value to both sides of the equation.
5. Factor the perfect square trinomial.
6. Take the square root of each side and solve.
Catherine Brito.
for 5c, when squaring both sides, doe the outside radical get squared as well?
hmmm… I don’t think you mean 5c, which is
…
How do you do problem 2) a. 4sqrt5xy^5 times -2xsqrt75x^2
you mean 1) a?
(4\sqrt{5xy^5})(-2\sqrt{75x^2})=-8x\sqrt{375x^3y^5}=-8x\sqrt{25}\sqrt{15}\sqrt{x^2}\sqrt{x}\sqrt{y^4}\sqrt{y}=-8x5xy^2\sqrt{15xy}=-40x^2y^2\sqrt{15xy}$
Ok I’ll admit I messed up on this equation on the first exam!
. We were supposed to express the solution using positive exponents only. Silly me, I multiplied the x^-2, y^2, and z by each of the variables in the second parentheses! I realized that’s not how you’re supposed to solve this equation, but my question is for the Zs, they cancel out right? Since positive Z and z^-1 should equal to 0.
Hi meyvin72 — you’re right,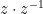
“cancel out” — the exponent becomes zero, and 
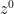equals 1.
(x^-2 y^2 z) \cdot (2x^-3 y^-1 z^-1)
(x^{-2} y^2 z) \cdot (2x^{-3} y^{-1} z^{-1})
$latex (x^{-2} y^2 z) \cdot (2x^{-3} y^{-1} z^{-1})
nice!
My internet was down! sorry
= 