Biodiversity and the Hudson River

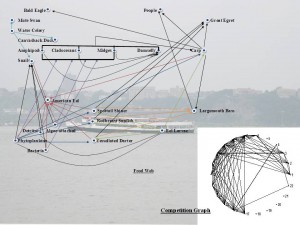
Flowing from the Lake Tear of the Clouds, North the Hudson River journeys 315 miles and drops 4,322 feet in elevation before emptying itself into New York Harbor. The Hudson River is home to diverse populations of fish, birds, and mammals that cohabit and compete among themselves for resources. Recently the American shad, Atlantic sturgeon, river herring (blue back herring and alewife), American eel, and largemouth bass are in decline. Intense economic harvesting pressure and overexploitation cause coastal and marine species to decline. Therefore, harvesting and fishing should be managed properly and carefully to avoid decline of current population. Food web analysis provides important information regarding the nature of competition among various organisms.

Cluster analysisin graph theory is a popular method to seek partition of a given data set into several clusters so that the data points within the same cluster are more similar than those belonged in the separate clusters. In this project we will use cluster analysis using the concepts of linear algebra to study the competition among various species in a given food web, in particular, competition among various Hudson River species. Students will find a partition of the competition graphs based on the Hudson River food web such that the strength of competition (for shared preys) between two clusters (two groups of predators) is as low as possible; however, the strength of competition within the same clusters is as high as possible.

Big Ideas behind this project

Study and analyze Hudson River Food Web and its competition graph to interpret the strength of species competition. Upon completion of this project, students should be able to answer the following questions:

• Which predator species are more connected than others?
• What happens if a specific species (particularly, a prey) dies out? Particularly, how does the removal of a particular species affect its predators and also the overall competition among all predator species?

I believe through this project students will gain some insights to the mechanisms of interactions and competition among various species. Students will be able to propose further measures for early intervention if any species dies out, share their knowledge, and create public awareness of the need to promote a healthy and balanced ecosystem in their own community.

My goals as the course instructor are:

• To assist students develop a deep understanding of core mathematical concepts and help them appreciate the usefulness of mathematics to analyze and explain their community and environment.
• To create challenging environment for high achiever students.
• To provide training in conducting research in an interdisciplinary field combining mathematics and ecology based on biodiversity of the Hudson River Estuary; a topic that is carefully chosen to hold students’ interests.
• To motivate students in higher studies in an interdisciplinary field.
• To help students retain knowledge for long term.

Students Learning Outcomes

• To solve systems of linear equations using matrices.
• To identify and use vector properties (spaces, subspaces, bases, inner product).
• To identify properties of matrices (inevitability, eigenvalues, eigenvectors).
• To use computer technology to solve practical problems.
• To learn how to collect data.
• To learn how to apply core mathematical concepts (particularly eigenvalues and eigenvectors) in solving real-world problems.
• To understand interdisciplinary approach and the significance of it in real-world applications.
• To write technical reports and disseminate the key findings.
• To understand how to present research findings.
• To learn how to work as a team.

How this will be done?

• Teach core concepts from Linear Algebra; emphasize usefulness of eigenvalues and eigenvectors.
• Require students to prove few theorems, lemmas and propositions regarding eigenvalues and eigenvectors.
• Require students to review literature.
• Introduce basic graph theory concepts and its applications to food web and species competitions.
• Require students to visit website and show them how data is collected.
• Require students to go to a Hudson River Fund seminar.
• Require students to verify few theorems using the Hudson River data.
• Introduce spectral clustering method.
• Require students to analyze the Hudson River competition graph using spectral clustering technique.
• Require students to interpret the results and make conclusions based on their findings.
• Require students to write a technical report or present their findings.
• Require students to produce fliers to be distributed later in high schools or local clubs in their own neighborhood.

Assessment

I will assess the course and the project outcome as follows:

• Individual student’s grading will be done based on a rubric. The rubric will be created based on learning objectives.
• Report and final presentation will be graded.
  

  FoodWeb and the Competition Graph

• Long term follow up will be carried out with interested students.
• A pre-test and post-test survey will be administered.