Your first OpenLab assignment is to introduce yourself to your classmates (and to me). This assignment is due Friday, Sept 20.
(Completing this assignment will earn you a point towards the participation component of your course grade. Late submissions will receive partial credit.)
Assignment: Write a comment in reply to this post (scroll to the bottom to find the “Leave a Reply” box–if you’re viewing this from the site’s homepage, you will need to click on the post’s title above, or click on the Comments link to the left):
In a brief paragraph (3-5 sentences), introduce yourself in whatever way you wish.
(What do you want your classmates to know about you? Some ideas: where you’re from, where you live now, where you went to high school, your major, your interests outside of school, etc. See the comments below to read introductions from people who have already posted.)





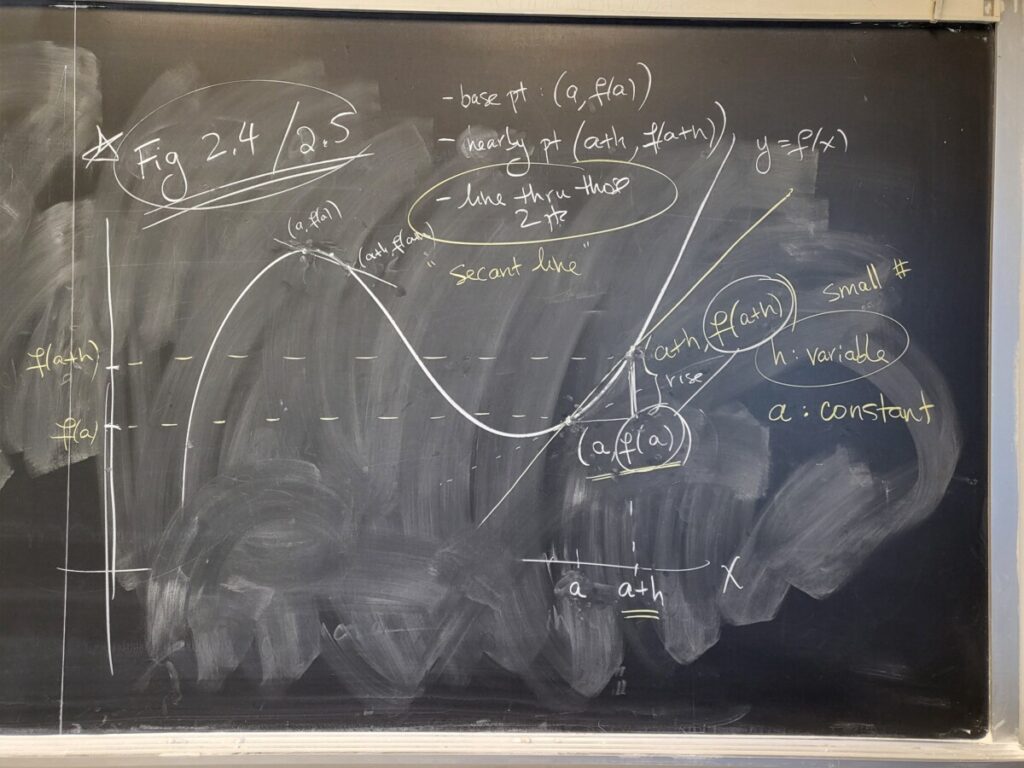
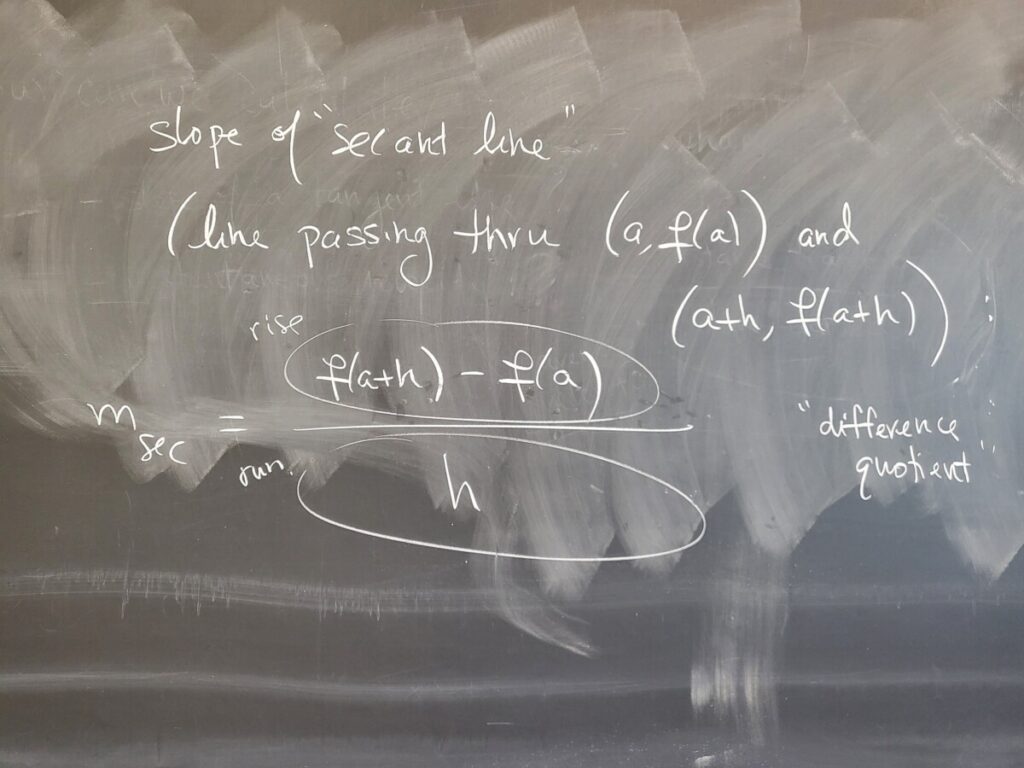


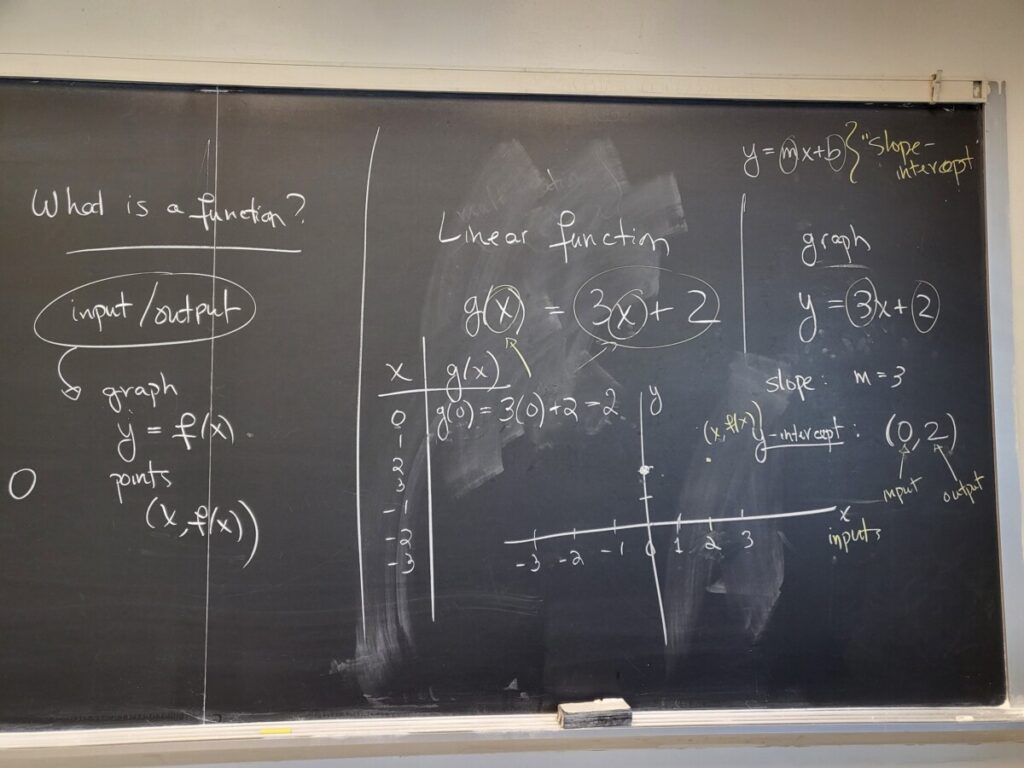






Recent Comments