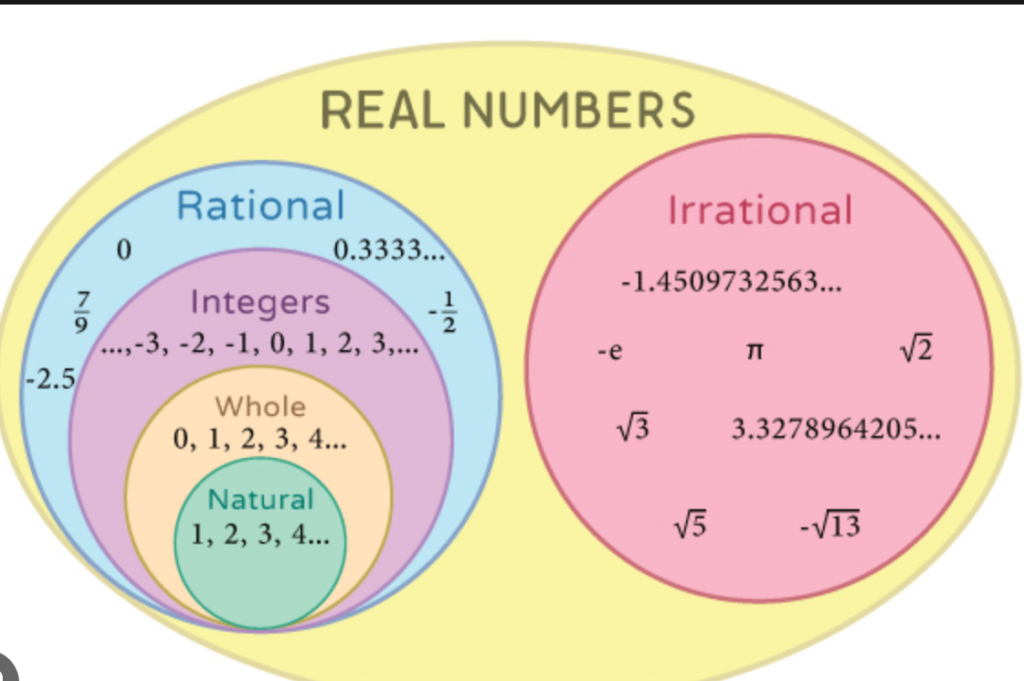
Having listened to the MEDU 3000 lecture on “What is a number?” I developed a fresh viewpoint on how to define a number. When describing a number’s significance, mathematicians use the term “real number,” donated by the symbol R. A horizontal line known as the number line contains all four major sorts of numbers. Any given point on the number line is considered a number. So what kind of numbers are shown on a number line? The number line consists of natural numbers, integers, rational and irrational numbers. The real number system consist of natural number’s in other words refers to “counting numbers,” consisting of only positive numbers (even or odd numbers.) Fun fact: A natural number is produced when two positive natural numbers are multiplied or added. Let’s try it out! 4,000+ 3,500 =7,500 or when multiplying you get 14 million! Natural numbers only include positive numbers, we cannot multiply or add negative numbers with natural numbers because it can result in a negative answer. The same natural numbers make up whole numbers, but they also include zero.
Integers are the next on the number line. Unlike natural numbers, integers include negative numbers, in addition to including positive numbers and zero. Integers can often be represented by the symbol Z. Another fun fact about integers, the addition, multiplication, and subtraction of any two integers result in an integer! Except for division, the solution can result in a decimal or remainder. We want to avoid a decimal or a fraction!
Thirdly, the number line consists of rational numbers. In simple words what defines a rational number is any number that can be written as a fraction a/b or a decimal: 0.5446, 0.48. The denominator and numerator share a relationship which is the presence of any integer number, except for zero in the denominator. Mathematically speaking, a numerator p and a non-zero denominator q. The most common application of rational numbers is in the measurement of various quantities, including mass, time, length, and other types that are described as rational number.
Fourthly, the number line consists of irrational numbers. A real number that is not rational, is called an irrational number. Irrational numbers, can’t be expressed as a fraction. For example we can consider pie, or any radical expression as a radical expression. So when do we use irrational numbers? How are irrational numbers different from other real numbers on the number line? Irrational numbers are used “indirectly,” it can be used to explain the measures found in nature. Trigonometric ratios, and Euler’s Number e, used in Logarithms and Algebra, need the use of irrational numbers.
All of the numbers I have discussed are regarded as real numbers. The real numbers on the number line are natural numbers, integers, rational, and irrational numbers. A number can be found at any place on the number line. Except for imaginary numbers, all numbers are real.
Demonstration of the Real Number System shown below.
Works cited



Leave a Reply