Category: Videos (Page 2 of 6)

Class Info

• Date: Mon Dec 4
• Meeting Info: 10a-11:40a, N719

Announcements

WebWork:

• “Series – Divergence Test” – due Wed Dec 6
• “Series – Comparison Tests” – due Wed Dec 13

Schedule for the rest of the semester:

I outlined the schedule for the rest of the semester:

• We have class tomorrow (Wed Dec 6) and Monday Dec 11, where we will cover additional topics on infinite series–please work on the WebWork sets on this material!
• Exam #3 will consist of a take-home component which will be distributed in class next Monday (Dec 11) and will due the following Monday (Dec 18)
• We do not meet on Wed Dec 13 (Tues Dec 12 and Wed Dec 13 are reading days, so no classes will meet)
• There will also be an in-class component which we will take that Monday Dec 18 (the take-home exericses will serve as preparation for the in-class exam); we will also review for the final exam that day
• The final exam is in-class on Wednesday Dec 20
• See the 1st board snapshot below for a day-by-day outline of the schedule

Topics

See the schedule on the left-hand side:

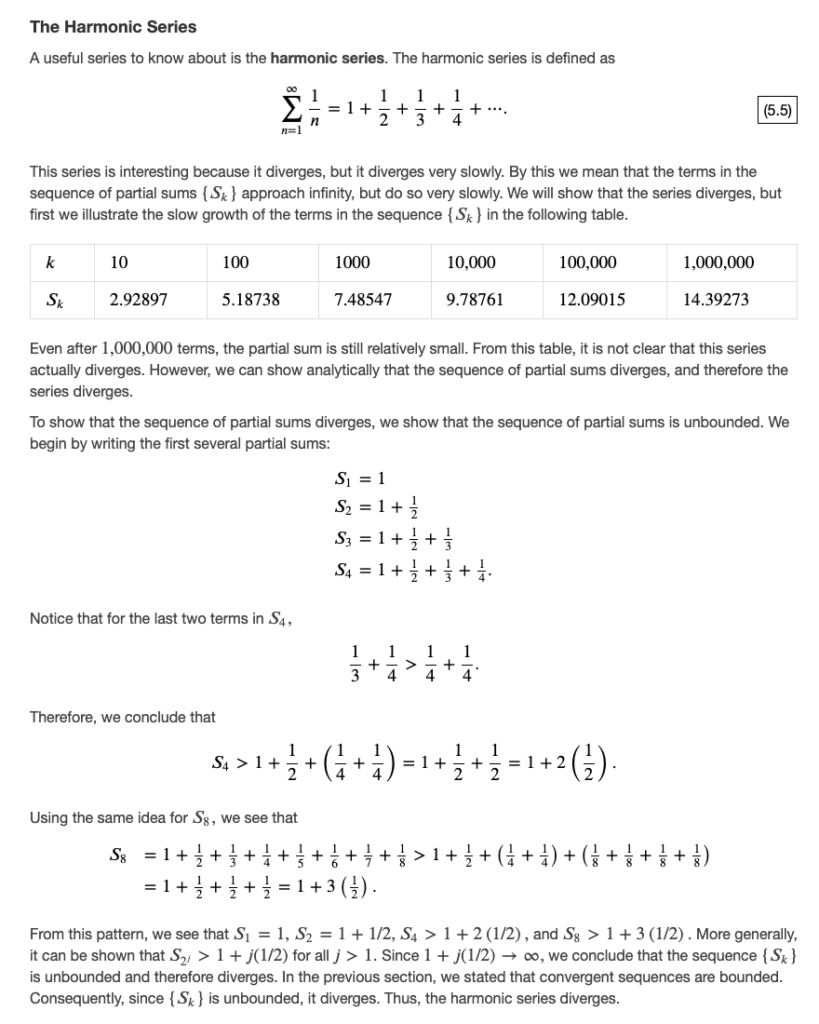
We briefly discussed the integral test, as a way of classifying “p-series”:

The integral test and p-series test are covered in Sec 5.3.

We then introduced the limit-comparison test, which is used to “compare” a given infinite series to one we know converges or diverges; the test consists of looking at the limit as n goes to infinity of the ratio a_n / b_n.

In this first example, we compared the given series (Sigma 1/(n^2+1) to the p-series with p=2, since they are very similar, and we know the latter converges:

Here is the presentation in the textbook (from Sec 5.4), which starts with a similar example:

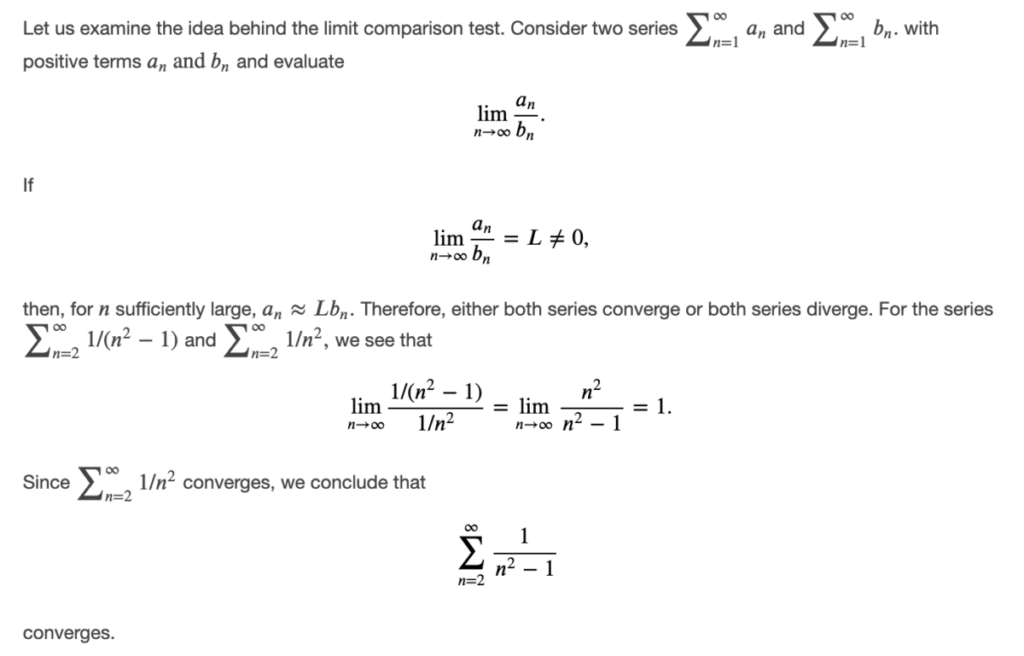
We then outlined two examples from the textbook:

We will do a couple more examples of the limit-comparison test tomorrow from the WebWork, then go on to discuss alternating series.

Class Info

• Date: Wed Nov 29
• Meeting Info: 10a-11:40a, N719

Announcements

WebWork:

• “Series – Infinite Series” – due Mon Dec 4
• “Series – Divergence Test” – due Wed Dec 6
• additional WebWorks on infinite series will be assigned as we cover the topics in class, so please keep up with the WebWork!

Topics

We summarized geometric series, and went through a couple more examples from “Series – Infinite Series” that involve a “sum rule” for infinite series:

We then introduced the Divergence Test (or “n-th term test”)–if the individual terms in the series do not go to zero, then clearly the series diverges! This is covered in Sec 5.3.

We applied it to examples of infinite series where the n-th term is a ratio of two polynomials of equal degree:

Finally, we discussed the harmonic series–this is the classic example of an infinite series where the individual terms do go to zero, but the series diverges!

We sketched one proof of divergence last week, with the integral test (which is covered in Sec 5.3, after the Divergence Test).

Another proof of divergence is by “grouping” terms in the series together, as shown in the textbook (Sec 5.2):

We looked briefly at the wikipedia page for the harmonic series, which includes the two different proofs of its divergence.