Class Info
- Date: Mon Oct 23
- Meeting Info: 10a-11:40a, N719
Announcements
WebWork:
- finish “Integration – Integration by Parts” (extended to Mon Oct 30)
- work on “Area Between Curves”
Topics
We went through one more examples from the WebWork “Integration – Partial Fractions”–a longer more challenging exercise, given that it involves “an irreducible quadratic factor” in the denominator (i.e., a quadratic that can’t be factored further–in this case “x^2 + 9”:

For such a factor, we have to use a linear factor numerator (“Bx+C”) above, and we have to use the “method of equating coefficients” to solve for B and C (since there’s no x-values which make the irreducible quadratic factor 0!):

The last steps, in order to integrate is to split up the numerator over the quadratic factor into two separate terms:

We can solve the first two integrals. But for the last integral–the constant over x^2+9–we need the following “inverse trig” integral:

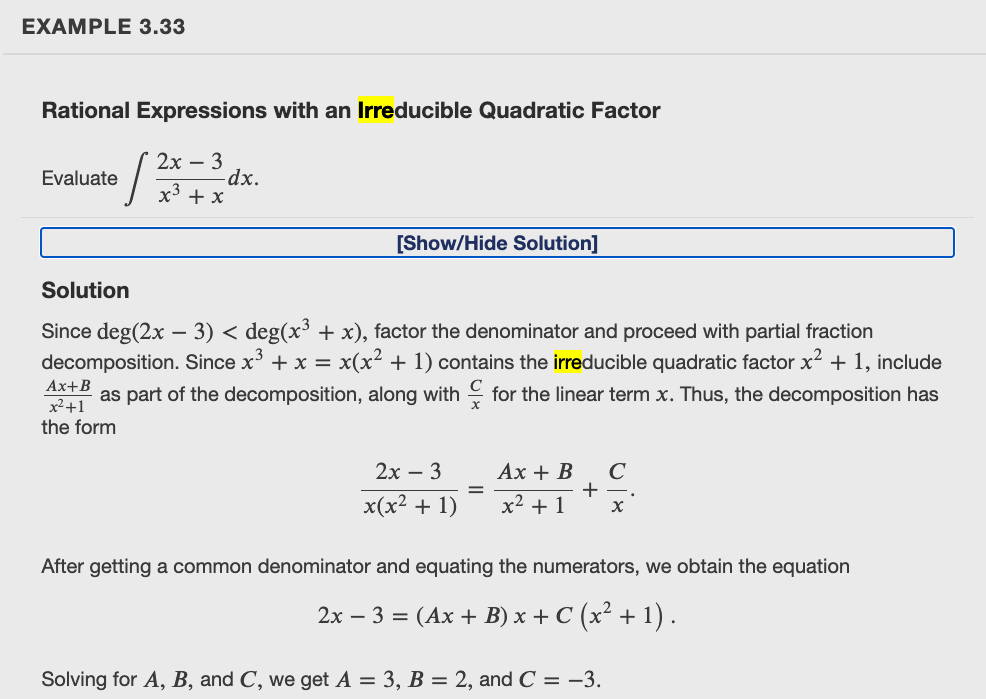
We mentioned that you can also study Example 3.33 Sec 3.4 of the textbook, which involves the (simpler) irreducible quadratic factor x^2+1:
We finished the class by starting to look at how to set up definite integrals to calculate “areas between curves”:

Here is a video with some similar examples:



Leave a Reply