Category: Project #1 groups (Page 1 of 2)

Section 4.2 Cooling and Mixing

Group members: 

Imerson Memko, Nelson Feng Feng, Timothy Khan, Bharat Dangi, Rafael Diaz, Keion Smith, Cristofer Martinez 

Lesson

The cooling and mixing section of the book is split into two lessons, for cooling and mixing respectively. Both of these sections consist of real world problems involving temperature, mixing of materials and time. 

Cooling problems consist of using a first order differential equation of the form,   

which if solved and rewritten will yield the form 

In these problems you need to be able to identify T0, Tm and t and then plug them into the formula. Most of the questions will ask you to find the temperature after a certain amount of time so you should be solving for t. Below are some examples of cooling problems from our group.

For mixing, most of the problems consist of mixing salt and water in a tank, draining the tank, and then determining the amount of salt that is left after a certain amount of time. For these problems we need to determine Q’ which is equal to the difference between the rate at which the salt enters the tank, and the rate at which it leaves the tank. Therefore:

To find the rate in you simply multiply the amount of salt poured in with the rate at which it’s being poured in so:

    rate in = (lb/gal) * (gal/min)

The rate out in the other hand you multiply the amount of salt already in the tank with the rate at which it’s being poured out so:

rate out = (concentration) *(rate of flow out)

rate out = (lb/gal) * (gal/min)

This can also be written as:

Q(t) / (concentration)*(rate of flow out)

You then rewrite Q’ and you should be left with the first order equation

Q’ = rate in – Q / rate out

and solve like any other first order equation that we have done.

Examples from group

Imerson Memko and Nelson Feng Feng Project Part 1

Timothy Khan and Bharat Dangi Project Part 1

Rafael Diaz Project Part 1

Keion Smith Project Part 1

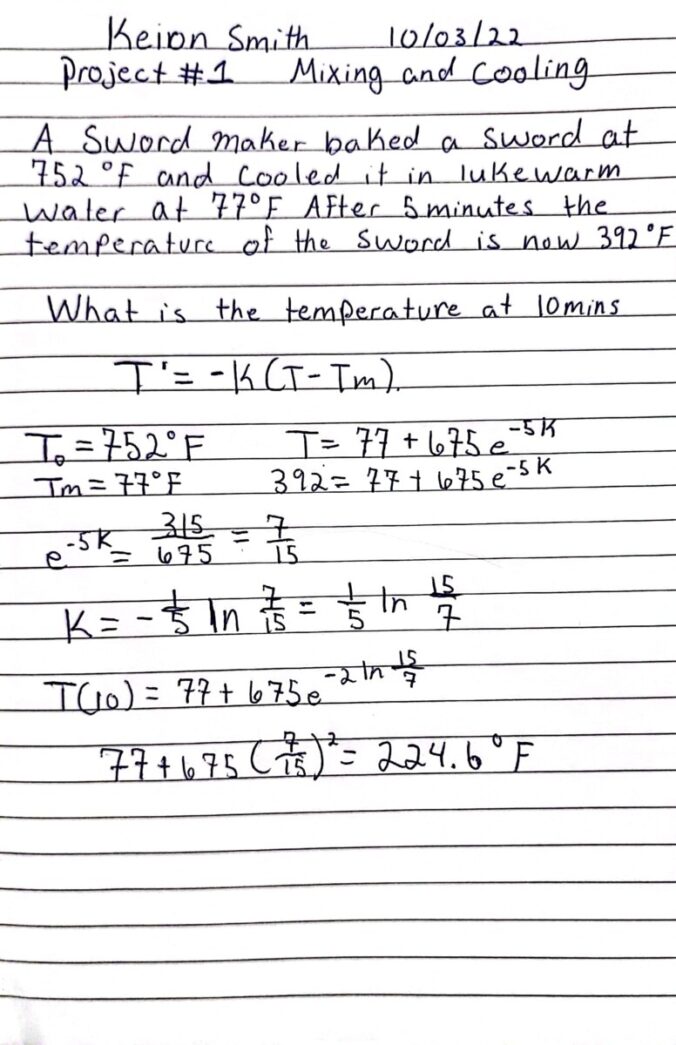
Keion Smith and Cristofer Martinez Project 1

Problems for the class

A man is holding a thermometer on a hot day in Brazil. The thermometer reads 90F. The man then teleports to Antarctica where the weather is 5F. After 1 minute it reads 50F. What will it read after 3 minutes?

A tank initially contains 50 pounds of sugar dissolved in 480 gallons of Kool-Aid. Starting and t0 = 0, Kool-Aid that contains ½ pounds of sugar per gallon is poured into the tank at a rate of 4 gal/min. The mixture is drained from the tank at a rate of 3gal/min. Find the amount of sugar Q(t) in the tank.

Part 1.a. The main concepts in mechanics are force, mass, and motion. Mass is a measure of a body to resist changes in its state of motion. Forces, on the other hand, accelerate bodies, which is to say, they change the state of motion of bodies to which they are applied. This ballet is the backbone of mechanics as we know it. In this lesson, we will focus on the implications that this complex relationship protests in Newton’s Second Law.

Part 1.b. Our group focused on math issues including basic mechanics. This included Newton’s Second Law of Motion, which states that the gravitational field’s force on an item is proportional to its mass and inversely proportional to the square of its distance from the center of Earth. The variables involved in our problems were the unit pound (lb.) a measurement of force, foot (ft) a measurement of length, feet per second squared (s/ft) a unit of acceleration, and foot per second (ft/s) a unit of both speed and velocity. Our exercises consisted of first order differential equations.  Where the equation dy/dx =f (x, y) with two variables x and y represents a first-order differential equation. It only contains the first derivative dy/dx, hence the equation is of first order and no higher-order derivatives exist. Our problem was solved using the variable separable method. The procedure included isolating the variables, integrating, and solving for the variables.

Part 2.a. LINKS: https://openlab.citytech.cuny.edu/https-openlabcitytechcunyedu-poiriermat2680fall2022/2022/09/28/project-1-part-1-section-4-3-problem-5-2/

https://openlab.citytech.cuny.edu/https-openlabcitytechcunyedu-poiriermat2680fall2022/2022/09/26/project-1/

Part 3.a.