Category: Project #1 (Page 2 of 6)

Part 1.a. The main concepts in mechanics are force, mass, and motion. Mass is a measure of a body to resist changes in its state of motion. Forces, on the other hand, accelerate bodies, which is to say, they change the state of motion of bodies to which they are applied. This ballet is the backbone of mechanics as we know it. In this lesson, we will focus on the implications that this complex relationship protests in Newton’s Second Law.

Part 1.b. Our group focused on math issues including basic mechanics. This included Newton’s Second Law of Motion, which states that the gravitational field’s force on an item is proportional to its mass and inversely proportional to the square of its distance from the center of Earth. The variables involved in our problems were the unit pound (lb.) a measurement of force, foot (ft) a measurement of length, feet per second squared (s/ft) a unit of acceleration, and foot per second (ft/s) a unit of both speed and velocity. Our exercises consisted of first order differential equations.  Where the equation dy/dx =f (x, y) with two variables x and y represents a first-order differential equation. It only contains the first derivative dy/dx, hence the equation is of first order and no higher-order derivatives exist. Our problem was solved using the variable separable method. The procedure included isolating the variables, integrating, and solving for the variables.

Part 2.a. LINKS: https://openlab.citytech.cuny.edu/https-openlabcitytechcunyedu-poiriermat2680fall2022/2022/09/28/project-1-part-1-section-4-3-problem-5-2/

https://openlab.citytech.cuny.edu/https-openlabcitytechcunyedu-poiriermat2680fall2022/2022/09/26/project-1/

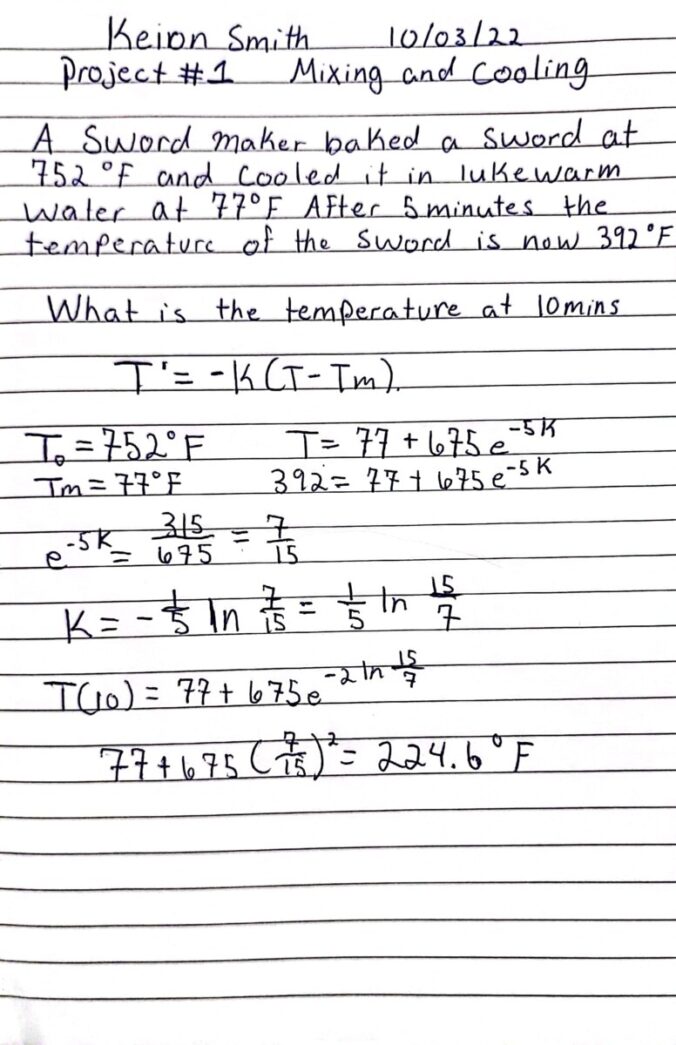
Part 3.a.