MAT1375 Precalculus Spring2014 MW

Topics: (to be expanded later when I have time)

• Inequalities and intervals and interval notation

• Solving absolute value inequalities – Note: We only used the first method given in the textbook for solving the inequalities. (See Example 1.17) This method can also be used for other types of inequalities (and we will be using it later on). Please avoid using the second method unless and until I have time to go over it in class, as many people do it wrong!

Homework:

• Add to or change your email address in CUNYFirst to an address that you usually check.

• If you do not regularly check your City Tech email, here are instructions about forwarding it to another email address, which I also handed out in class. (You can also use Mail.app on Macs, or MS Outlook, to POP or iMAP the emails, if you use either of those two programs already.)

• Register in OpenLab and join this course, using the instructions on the handout. (Or see here.) Registering will give you more access to features in OpenLab. Joining the course means you will automatically be notified when there are new posts, among other things.

• Log in to WeBWorK following the instructions here and start working on the Orientation assignment (at least the first few problems). Then do the other assignment.(This time it is due 11 PM Tuesday, in case there are problems logging in.)

• Please see the First day’s post and make sure that you do or did everything there!

• Routine homework: in Session 1, Exercises  1.6, 1.7 (a)-(f) but skip (e) for now

• Read ahead Session 2

• Warmup for Functions (due 11 PM Sunday):  make sure you do the Warm-Up from last time as well! (If you already did it, you should not do it again.)

Signing on to WeBWorK:

• Go to the course blog in OpenLab and look for today’s post. There will be a link on each day’s post when there is WeBWorK homework due.

Or you can go directly to mathww.citytech.cuny.edu/webwork2. However, be aware that you are required to check the course blog on a regular basis for details about all homework and other information.

• Click on the link MAT1375-Shaver-MW or MAT1375-Shaver-TTh, according to the days your section meets.

• in the login screen, enter your login name and password. Your initial login and password are the same thing: your first initial followed by your last name, all lower-case. [For example, my login is sshaver.]

• Very important: Once you are logged in, go to the Password/Email link on the left side of the page under “Main Menu”’ and change your password

• Also enter an email address that will be used to contact you or reply to questions you ask while using WeBWorK. Please use an address that belongs to you only.

Once you have completed the steps above, look at the Orientation assignment. When you open the assignment, look over the page carefully. Look first at the information given in the box at the right side of the page. Then you can start working on the problems. This assignment is designed to familiarize you with WeBWorK and how you enter your answers. You can keep playing with this Orientation assignment throughout the semester whenever you feel the need.

When you have some familiarity with WeBWorK, go on to the regular assignment “AbsoluteValueEquationsInequalities” Please note that it is due not later than 11:00 PM of the evening before class. I am giving one week for this first assignment only, in case there are any problems with logins. However, usually, the assignments will always be due 11 PM the night before the next class meeting after they are assigned. Start working well in advance of the due date and time so that you can have time to redo problems whose answers are incorrect, and so that you will not have to rush. (Please consult the course information sheet for policy on extensions.)

You may usually resubmit a new answer to a problem if you get it wrong. If you need to redo any problem, you can and should consult your notes, the textbook, discuss with another student, post questions to the blog, etc. before you submit a new answer. Note that usually each student will receive a different version of the problem, although they will be similar.

At the bottom of each assignment is a button you can use to email me if you encounter any difficulties using WeBWorK or if you believe that your answers are correct but they are being marked wrong. Please do not use this to ask how to do a problem: rather, just for questions related to WeBWorK itself.

Welcome to your MAT 1375 class site on OpenLab.

If you have not already got a copy of the textbook, it is available here.

The topics for today:

• the Real Number system. It is background to what we do in the rest of the chapter (and the rest of the course!)

First we should understand very clearly that certain words used in Mathematics (or any technical subject) may be the same as words used in everyday talk, but in Mathematics these words have specific meanings which are often different from (but sometimes related to) the meanings that they have in everyday talk. In this case, “real” is just a name for this set of numbers: they are not more “real” in the everyday sense than any other set of numbers. And also, “number” in everyday talk almost always means what is called in mathematics a “natural number”.  So we have to be careful that we use the words appropriate to the context!

The sets of numbers we considered: (see in the textbook for notation)

• The natural numbers (also called the counting numbers) 1, 2, 3, 4, …

• The whole numbers – these are not mentioned in Session 1 in the textbook, but it is a name we use a lot so you should know what they are. The whole numbers are just the natural numbers along with 0: 0, 1, 2, 3, 4, …

Be aware that some people use “whole number” as a synonym for “natural number” though!

• The rational numbers: please note that these are numbers which can be expressed as a ratio (or quotient) of two integers: they do not have to come to you already in this form. Thus 33 is a rational number because you can write it as 33/1 (for example, or 66/2, or -66/(-2), etc.)

We mentioned that the denominator cannot be 0: the fact that division by 0 is impossible is rather important, and you can benefit by thinking carefully about it. In a sense, all of Calculus is concerned with this question!

• The real numbers: they are the points on the number line, interpreted as directed distances away from 0. Once you have designated a point to be 0 and another point to the right of it to be 1, that determines your unit of measurement and you can mark off all the other points using it (or fractions of it: it is a little mysterious how the irrational numbers are to be found though!) By saying “directed distances” I mean that the distance is counted as positive if the number is to the right of 0, and negative if the number is to the left of 0. (Normally distances are always positive.) This is the first instance of something we will later be calling vectors, which are lengths which also have direction.

• We noted that there are points (lengths) on the number line which are not rational numbers. The most famous example of this is the square root of 2. This is most definitely a length, since it is the length of the hypotenuse of a right triangle whose sides are both 1. (Use the Pythagorean Theorem and prove this to yourself!) However, the square root of 2 cannot be expressed as a ratio of integers.  You can read the proof here.

There are many other real numbers which are not rational numbers. For example, pi, e (the base of the natural logarithms), and the square roots of any number which is not a perfect square, are all irrational. In fact, there are more irrational numbers than rational numbers. Weird!

• The absolute value of a real number. There are two ways of defining the absolute value: one is algebraic, and one is geometric.

Algebraic definition: for any real number ,

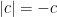 if  ,

 if  .

(This defines the absolute value as a piecewise-defined function: we will return to these in a later Session!)

Geometric definition: 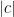is the distance from 0 to on the number line. (A distance is always non-negative.)

• Solving absolute value equations (see examples 1.4 – 1.9 in the textbook): notice that the first step is always to get rid of the absolute value sign by splitting the equation into two. (This is if the right-hand side is a positive number: what if the right-hand side is 0 or negative?)

Homework:

• Reread/review the material covered in class.

• Routine Homework problems: Session 1, Exercises 1.1, 1.2, 1.3, and 1.4 (the assigned parts: see the course outline). If you have questions about the homework problems or anything else related to this material you can post them as comments to this post. (Later we will be using an online discussion tool called Piazza.)

• Read Session 1 in the textbook. You may also want to read  ahead the start of Session 2.

• Follow this link to the Warm-Up: use it to send me  your answers to Exercise 1.1 so I can check them for you. Please use your imagination and do some thinking or research while answering these questions! I will post all the responses (without names or IDs) after the deadline of 11 PM Sunday the 2nd of February.

Please note that questions with red asterisks beside them are required. (In this case, all of them.) Your ID number is the EMPLID from CUNYFirst.

Note: normally, Warm-Ups are due by 11 PM the night before the next class meeting. I am giving an extra class meeting for this first one only.