1. $\rhd$ Adding and Subtracting rational expressions with like denominators (3:45) This video contains one example of adding and one of subtracting rational expressions, namely:
[Example 1] $\dfrac{6}{2x^2-7} + \dfrac{-3x-8}{2x^2-7} = \dfrac{-2-3x}{2x^2-7}$
[Example 2] $\dfrac{9x^2 +3}{14x^2 – 9} – \dfrac{-3x^2+5}{14x^2 – 9} = \dfrac{12x^2 – 2}{14x^2 – 9}$
2. $\star$ Practice: Adding and subtracting rational expressions with like denominators. Problems similar to: 
3. $\rhd$ Adding rational expressions with unlike denominators (2:43) $ \dfrac{a}{b} + \dfrac{c}{d}$.
4. $\star$ Practice: Adding rational expressions with unlike denominators. Problems similar to $\dfrac{8}{x+2}-\dfrac{6}{x+5}$. (4 problems)
5. $\rhd$ Adding rational expressions: unlike denominators (5:11) Problems such as $\dfrac{5x}{2x-3} + \dfrac{-4x^2}{3x+1} = \dfrac{-8x^3 + 27x^2 +5x}{(2x-3)(3x+1)}$.
6. $\rhd$ Subtracting rational expressions: unlike denominators (4:47) Solving $\dfrac{-5x}{8x+7} – \dfrac{6x^3}{3x+1} = \dfrac{-48x^4 – 42x^3 – 15x^2 – 5x}{(8x+7)(3x+1)}$.
7. $\star$ Practice: Adding rational expressions with unlike denominators. Problems such as $\dfrac{9}{x-7} + \dfrac{3}{x}$. (4 problems)
8. $\rhd$ Finding the least common multiple of two integers (4:15)
[Example 1] Find the $\mathrm{lcm}$ of 36 and 12.
[Example 2] Find the $\mathrm{lcm}$ of 12 and 18 (uses the prime factorization method).
9. $\rhd$ Finding the least common multiple with repeating factors (2:34) Find the $\mathrm{lcm}(30,25)$.
10. $\star$ Practice: Find the least common multiple of two integers. Find the $\mathrm{lcm}(6,10)$. (4 problems)
11. $\rhd$ Finding the least common multiple of polynomials (6:51)
Finds the least common multiple of $3z^3-6z^2-9z$ and $7z^4 + 21z^3 +14z^2$.
12. $\rhd$ Subtracting rational expressions (4:48)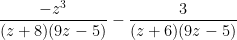 
13. $\star$ Practice: Combine the rational expressions. Problems such as $ \dfrac{9}{x^2-12x+36} + \dfrac{x}{x^2-36}.$ (4 problems)
