series – 2020 Spring – MAT 1375 Precalculus

Hi everyone! Read through the material below, watch the videos, and send me your questions. Don’t forget to complete the Daily Quiz (below this post) before midnight to be marked present for the day.

Lesson 24: The geometric series

Lesson Date: Tuesday, May 12th.
Topic: This lesson covers Chapter 24: The geometric series
WeBWorK: There are two WeBWorK assignments on today’s material, due in one week:
Sequences – Geometric
Series – Geometric

Question of the day: Can we add up infinitely many numbers?

Lesson NOtes (Notability – pdf):

This .pdf file contains most of the work from the videos in this lesson. It is provided for your reference.

Lesson-24_-The-geometric-series Download

Finite geometric series

Today we look at a new kind of sequence, called a geometric sequence, and the corresponding series, geometric series.

A geometric sequence is a sequence for which we multiply by a constant number to get from one term to the next, for example:

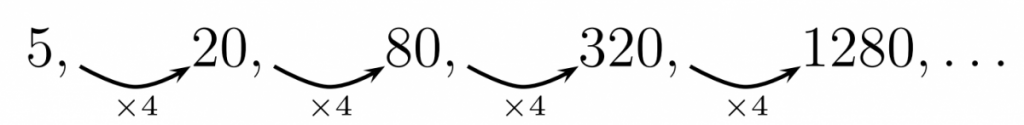
Definition 24.1. A sequence ${a_{n}}$ is called a geometric sequence, if any two consecutive terms have a common ratio $r$ . The geometric sequence is determined by $r$ and the first value $a_{1}$ . This can be written recursively as:
$a_{n} = a_{n - 1} \cdot r for n \geq 2$

Alternatively, we have the general formula for the $n$ th term of the geometric sequence:
$a_{n} = a_{1} \cdot r^{n - 1}$

Example 24.2. Determine if the sequence is a geometric or arithmetic sequence, or neither or both. If it is a geometric or arithmetic sequence, then find the general formula.
a) $3, 6, 12, 24, 48, \dots$
b) $100, 50, 25, 12.5, \dots$
c) $700, - 70, 7, - 0.7, 0.07, \dots$
d) $2, 4, 16, 256, \dots$
e) $3, 10, 17, 24, \dots$
f) $- 3, - 3, - 3, - 3, - 3, \dots$
g) $a_{n} = {(\frac{3}{7})}^{n}$
h) $a_{n} = n^{2}$

VIDEO: Introduction to geometric sequences, Example

Example 24.3. Find the general formula of a geometric sequence with the given property
a) $r = 4,$ and $a_{5} = 6400$
b) $a_{1} = \frac{2}{5},$ and $a_{4} = - \frac{27}{20}$
c) $a_{5} = 216, a_{7} = 24,$ and $r$ is positive

VIDEO: Finding the formula of a geometric sequence – Example 24.3

Example 24.4. Consider the geometric sequence $a_{n} = 8 \cdot 5^{n - 1},$ that is the sequence:
$8, 40, 200, 1000, 5000, 25000, 125000, \dots$

Find the sum of the first 6 terms of this sequence
$8 + 40 + 200 + 1000 + 5000 + 25000 = 31248$

VIDEO: Sum of a geometric series – intro example

Observation 24.5. Let ${a_{n}}$ be a geometric sequence, whose $n$ th term is given by the formula $a_{n} = a_{1} \cdot r^{n - 1} .$ We furthermore assume that $r \neq 1 .$ Then, the sum $a_{1} + a_{2} + \dots + a_{k - 1} + a_{k}$ is given by
$\sum_{i = 1}^{k} a_{i} = a_{1} \cdot \frac{1 - r^{k}}{1 - r}$

Example 24.6. Find the value of the geometric series.
a) Find the sum $\sum_{n = 1}^{6} a_{n}$ for the geometric sequence $a_{n} = 10 \cdot 3^{n - 1}$
b) Determine the value of the geometric series: $\sum_{k = 1}^{5} {(- \frac{1}{2})}^{k}$
c) Find the sum of the first 12 terms of the geometric sequence
$- 3, - 6, - 12, - 24, \dots$

VIDEO: The sum of a finite geometric series, Example 24.6

Infinite geometric series

Sometimes it makes sense to add up not just a finite number of terms in a sequence, but ALL the terms (infinitely many!).

Example 24.7. Consider the geometric sequence
$1, \frac{1}{2}, \frac{1}{4}, \frac{1}{8}, \frac{1}{16}, \dots$
What is the initial term? What is the common ratio?
Let’s try adding up some of the terms. Try this by hand, and by using the formula for finite geometric series. What happens if we add up ALL the terms?

VIDEO: Infinite geometric series – intro example and formula

Definition 24.8. An infinite series is given by the formula
$\sum_{i = 1}^{\infty} a_{i} = a_{1} + a_{2} + a_{3} + \dots$

Observation 24.9. Let $Missing or unrecognized delimiter for \left$ be a geometric sequence with $a_{n} = a_{1} \cdot r^{n - 1}$ Then the infinite geometric series is defined whenever $- 1 < r < 1$ . In this case, we have:
$\sum_{i = 1}^{\infty} a_{i} = a_{1} \cdot \frac{1}{1 - r}$

Example 24.10. Find the value of the infinite geometric series.
a) $\sum_{j = 1}^{\infty} a_{j},$ for $a_{j} = 5 \cdot {(\frac{1}{3})}^{j - 1}$
b) $\sum_{n = 1}^{\infty} 3 \cdot (0.71)^{n}$
c) $500 - 100 + 20 - 4 + \dots$
d) $3 + 6 + 12 + 24 + 48 + \dots$

Example 24.11. Consider the real number given by $0.555555 \dots$ . Rewrite this number as an infinite geometric series. Can you figure out what fraction it is equal to?

VIDEO: Infinite geometric series – examples

Lesson 23: Sequences and series

Lesson Date: Thursday, May 7th.
Topic: This lesson covers Chapter 23: Sequences and series
WeBWorK: There are three WeBWorK assignments on today’s material, due in one week:
Sequences – Introduction
Sequences – Arithmetic
Series – Finite Arithmetic

Question of the day: What is a sequence? What is a series?

Answer of the day: A sequence is just a list of numbers. A series is list of numbers, added up.

Lesson NOtes (Notability – pdf):

This .pdf file contains most of the work from the videos in this lesson. It is provided for your reference.

Lesson-23_-Sequences-and-series Download

Introduction to sequences and series

Today we will introduce two very powerful ideas (they are the building blocks of Calculus) – however, the ideas themselves are not complicated: sequences and series. They both have to do with lists of numbers, rather than individual numbers.

Definition 23.1. A sequence is an ordered list of numbers (we call each number in the list a term in the sequence). We write a sequence in order as follows: $a_{1}, a_{2}, a_{3}, a_{4}, \dots$
In short we write the above sequence as ${a_{n}}$ or ${a_{n}}_{n \geq 1}$

Let’s look at examples of some sequences. As you look at this example, keep the following questions in mind:

Is there a pattern in the sequence?
Can you predict what the next term of the sequence will be?
Can you predict what the 10th term of the sequence will be? What about the 20th, or the 100th?
Can you find a formula for $a_{n}$ , the $n$ th term of the sequence?

Example 23.2. Here are some examples of sequences.
a) $4, 6, 8, 10, 12, 14, 16, 18, \dots$
b) $1, 3, 9, 27, 81, 243, \dots$
c) $+ 5, - 5, + 5, - 5, + 5, - 5, \dots$
d) $1, 1, 2, 3, 5, 8, 13, 21, 34, 55, \dots$
e) $5, 8, - 12, 4, 5.3, 7, - 3, \sqrt{2}, 18, \frac{2}{3}, 9, \dots$

Example 23.3. Consider the sequence ${a_{n}}$ with $a_{n} = 4 n + 3$ . Calculate the first five terms of the sequence $a_{1}, a_{2}, a_{3}, a_{4},$ and $a_{5}$ . What is the $200$ th term of the sequence?

VIDEO: Introduction to sequences – Examples 23.2 and 23.3

Example 23.4. Find the first 6 terms of each sequence.
a) $a_{n} = n^{2}$
b) $a_{n} = \frac{n}{n + 1}$
c) $a_{n} = (- 1)^{n}$
d) $a_{n} = (- 1)^{n + 1} \cdot 2^{n}$

Another way to describe a sequence is by giving a recursive formula for the $n$ th term $a_{n}$ in terms of the lower terms. Here are some examples.

Example 23.6. Find the first $6$ terms in the sequence described below.
a) $a_{1} = 4,$ and $a_{n} = a_{n - 1} + 5$ for $n > 1$
b) $a_{1} = 3,$ and $a_{n} = 2 \cdot a_{n - 1}$ for $n > 1$
c) $a_{1} = 1, a_{2} = 1,$ and $a_{n} = a_{n - 1} + a_{n - 2}$ for $n > 2$

VIDEO: Two ways of describing sequences -Examples 23.4 and 23.6

Definition 23.8. A series is a sum of terms in a sequence. We denote the sum of the first $k$ terms in a sequence with the following notation:
$\sum_{i = 1}^{k} a_{i} = a_{1} + a_{2} + \dots + a_{k}$
The summation symbol $\sum$ comes from the greek letter $Σ$ , pronounced “sigma,” which is the greek letter for “S.”

Example 23.9. Find the sum.
a) $\sum_{i = 1}^{4} a_{i},$ for $a_{i} = 7 i + 3$
b) $\sum_{j = 1}^{6} a_{j},$ for $a_{n} = (- 2)^{n}$
c) $\sum_{k = 1}^{5} (4 + k^{2})$

VIDEO: Intro to series – Example 23.9

The arithmetic sequence

Definition 23.10. A sequence $Missing or unrecognized delimiter for \left$ is called an arithmetic sequence if any two consecutive terms have a common difference $d$ . The arithmetic sequence is determined by $d$ and the first value $a_{1}$ . This can be written recursively as:
$a_{n} = a_{n - 1} + d for n \geq 2$
Alternatively, we have the general formula for the $n$ th term of the arithmetic sequence
$a_{n} = a_{1} + d \cdot (n - 1)$

Example 23.11. Determine if the sequence is an arithmetic sequence. If so, then find the general formula for $a_{n}$ .
a) $7, 13, 19, 25, 31, \dots$
b) $13, 9, 5, 1, - 3, - 7, \dots$
c) $10, 13, 16, 20, 23, \dots$
d) $a_{n} = 8 \cdot n + 3$

VIDEO: Arithmetic sequences – Example 23.11

Example 23.12. Find the general formula of an arithmetic sequence with the given property.
a) $d = 12,$ and $a_{6} = 68$
b) $a_{1} = - 5,$ and $a_{9} = 27$
c) $a_{5} = 38,$ and $a_{16} = 115$

VIDEO: Arithmetic sequences – finding a general formula, Example 23.12

Example 23.13. Find the sum of the first 100 integers, starting from 1 . In other words, we want to find the sum of $1 + 2 + 3 + \dots + 99 + 100$ .

VIDEO: Arithmetic series – intro example 23.13

Observation 23.14 . Let ${a_{n}}$ be an arithmetic sequence, whose $n$ th term is given by the formula $a_{n} = a_{1} + d (n - 1)$ . Then, the sum $a_{1} + a_{2} + \dots + a_{k - 1} + a_{k}$ is given by adding $(a_{1} + a_{k})$ precisely $\frac{k}{2}$ times:
$\sum_{i = 1}^{k} a_{i} = \frac{k}{2} \cdot (a_{1} + a_{k})$

Example 23.15. Find the value of the arithmetic series.
a) Find the sum $a_{1} + \dots + a_{60}$ for the arithmetic sequence $a_{n} = 2 + 13 (n - 1)$
b) Determine the value of the sum: $\sum_{j = 1}^{1001} (5 - 6 j)$
c) Find the sum of the first 35 terms of the sequence
$4, 3.5, 3, 2.5, 2, 1.5, \dots$

VIDEO: Arithmetic series – finding the sum, Example 23.15

Tag: series

Lesson 24: The geometric series

Lesson NOtes (Notability – pdf):

Finite geometric series

Infinite geometric series

Lesson 23: Sequences and series

Lesson NOtes (Notability – pdf):

Introduction to sequences and series

The arithmetic sequence

The OpenLab at City Tech:A place to learn, work, and share

Support

Accessibility

Copyright

Creative Commons

The OpenLab at City Tech:A place to learn, work, and share

Support

Accessibility

Copyright

Creative Commons