Category: Series (Page 1 of 4)

Today’s quiz consisted of a single problem: find the fifth-degree Taylor Polynomial approximation of the function $f(x)=\ln (x)$ centered at $x=1$. In setting up for this Taylor Polynomial, we create a table with rows for all values of $n$ starting at $n=0$ and ending at the fifth-degree: $n=5$.

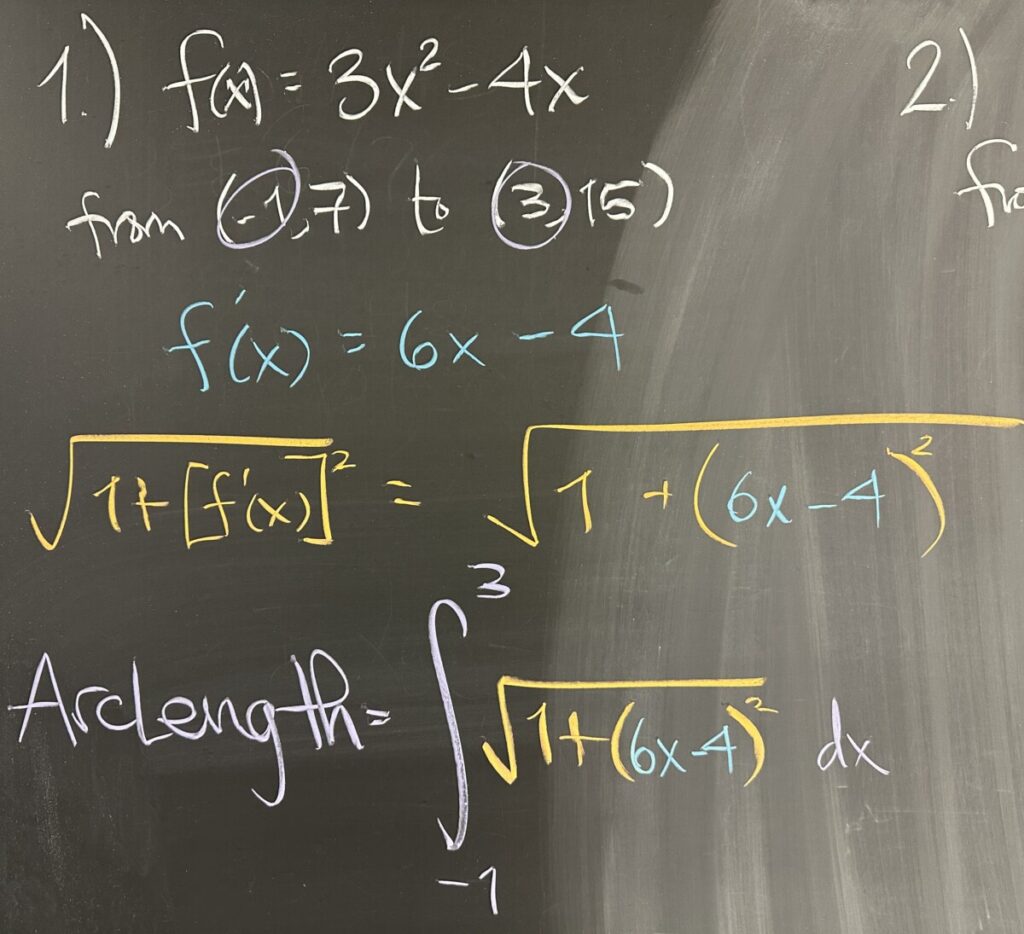
Today’s quiz contained three questions about arc length. The entire problem consisted of setting up (but not evaluating) the integral for arc length of the given functions. Recall that the arc length of $f(x)$ from $x=a$ to $x=b$ is calculated using the integral: $$L={\int }_a^b\sqrt{1+{[f^{\prime }(x)]}^2}dx$$

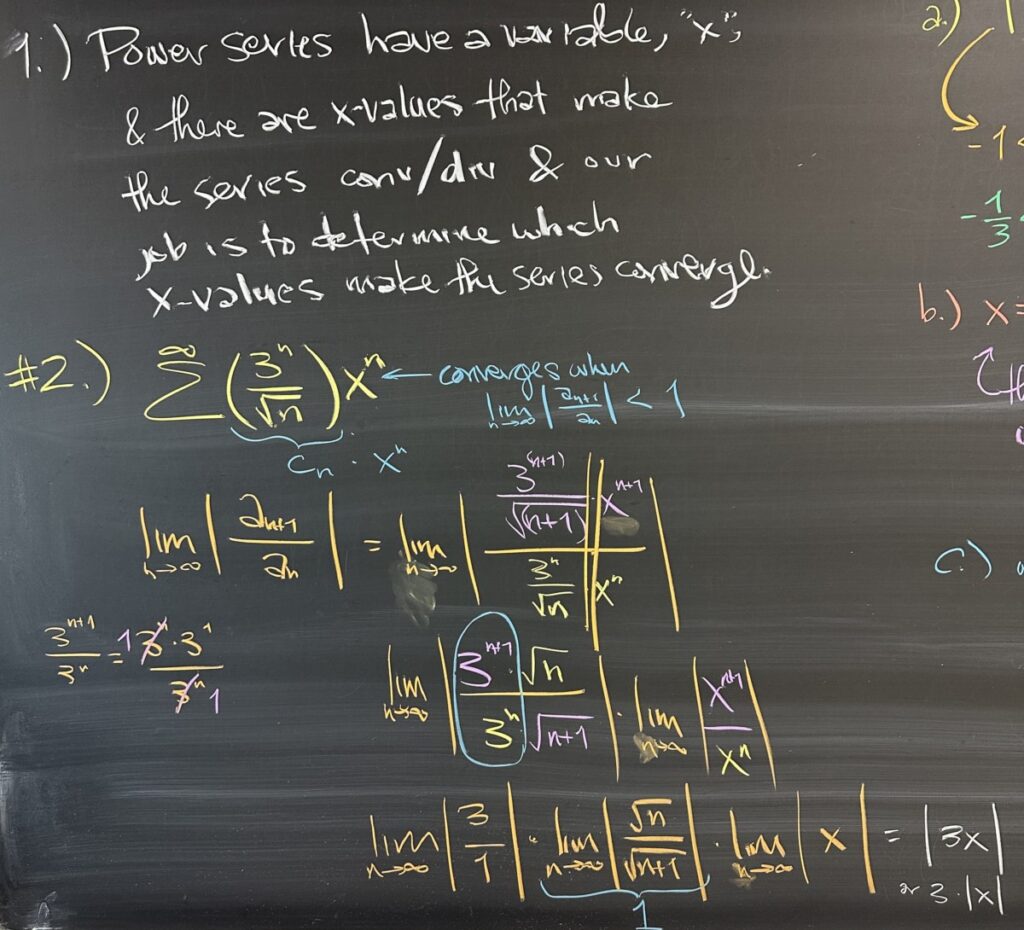
After today’s quiz, we started by talking about what makes “power series” different from the series that we’ve been working with so far this semester. The presence of $x$ as a variable in the series means that we have a series that can both converge AND diverge — depending on the value of $x$.