Tag: ti-84

Hi everyone! Read through the material below, watch the videos, and send me your questions. Don’t forget to complete the Daily Quiz (below this post) before midnight to be marked present for the day.

Lesson Date: Tuesday, April 21st.

Topic: This lesson covers Chapter 19: Inverse trigonometric functions.

WeBWorK: There is one WeBWorK assignment on today’s material, due next Tuesday 4/28:

Trigonometry – Inverse Functions

Question of the Day: Are the trigonometric functions $\sin(x),\cos(x)$ and $\tan(x)$ one-to-one functions?

The functions $\sin^{-1}, \cos^{-1},\tan^{-1}$

In this section, we are interested in the inverse functions of the trigonometric functions $y=\sin(x), y=\cos(x),$ and $y=\tan(x)$. You may recall from our work earlier in the semester that in order for a function to have an inverse, it must be one-to-one (or pass the horizontal line test: any horizontal line intersects the graph at most once).

The function $\tan^{-1}(x)$

Recall the graph of the function $y=\tan(x)$:

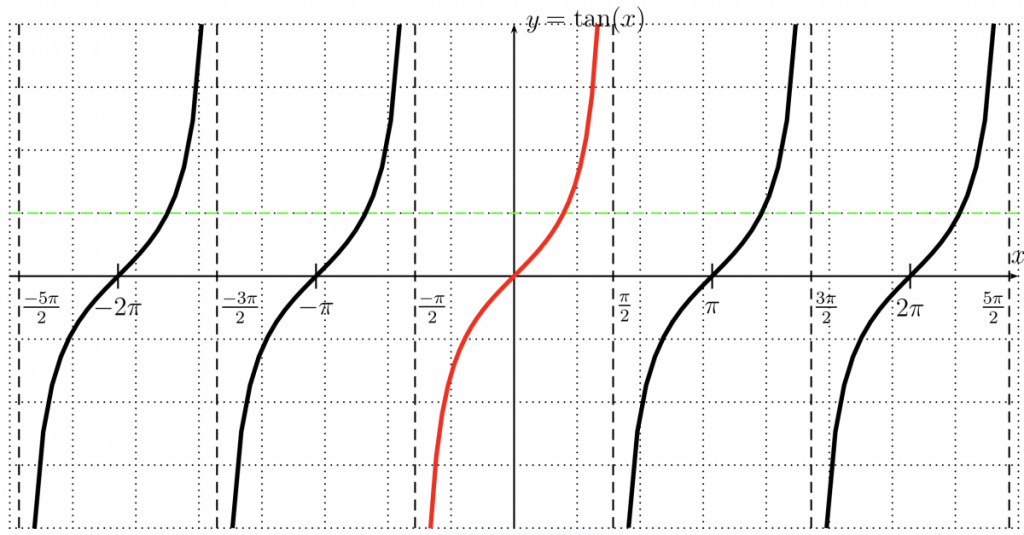
Notice that since the graph consists of a repeating pattern of vertical stripes, any horizontal line will touch the graph in multiple places – this graph FAILS the horizontal line test (it is NOT one-to-one). How can we define the inverse? By restricting the domain – that is, only looking at one of the repeating vertical stripes. If we only look at the part of the graph between $-\frac{\pi}{2}$ and $\frac{\pi}{2}$ then the function is one-to-one (that it, the red part of the function above is, by itself, one-to-one).

Definition 19.1. The inverse of the function $y=\tan (x)$ with restricted domain $D=\left(\frac{-\pi}{2}, \frac{\pi}{2}\right)$ and range $R=\mathbb{R}$ is called the inverse tangent or arctangent function. It is denoted by:
$y=\tan ^{-1}(x) \quad$ or $\quad y=\arctan (x) \quad \Longleftrightarrow \quad \tan (y)=x, \quad y \in\left(-\frac{\pi}{2}, \frac{\pi}{2}\right)$

Note that the inverse tangent function is written both $\tan^{-1}(x)$ and $\arctan(x)$ — they mean the same thing.

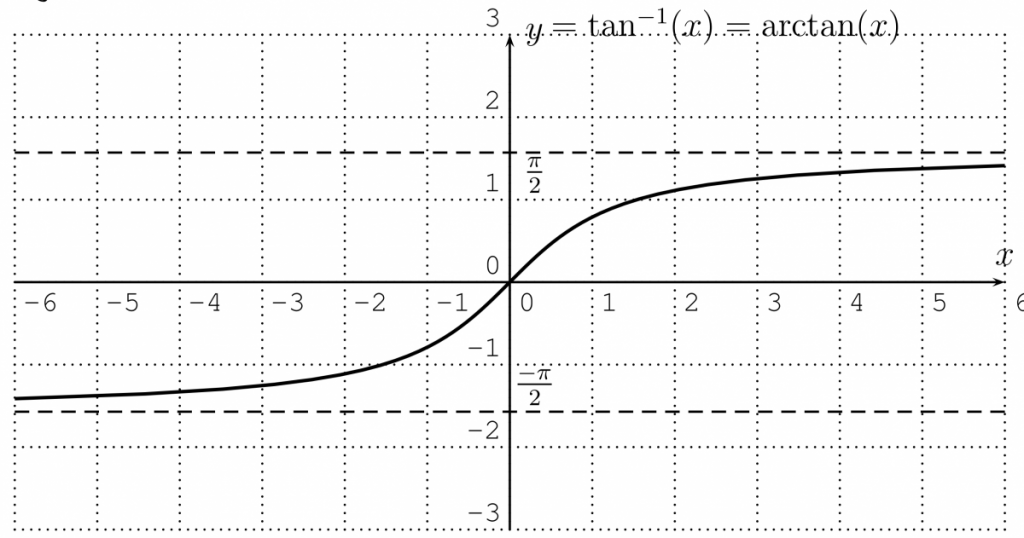
Observation: The inverse tangent is an odd function, so $\tan^{-1}(-x)=-\tan^{-1}(x)$
(recall that a function $f(x)$ is odd provided $f(-x)=-f(x)$)

Example 19.1 Recall the exact values of the tangent function from Chapter 17:

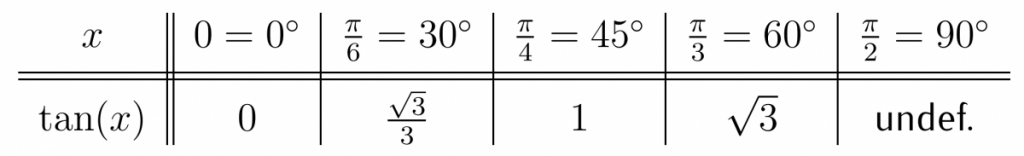
Use the table and Observation above to find exact values of the inverse tangent function. Give answers in both degrees and radians.
a. $\arctan(1)$ b. $\arctan\left(-\frac{\sqrt{3}}{3}\right)$. c. $\tan^{-1}(0)$

THE FUNCTION $\sin^{-1}(x)$

Consider the graph of the function $y=\sin(x)$. It is not one-to-one either:

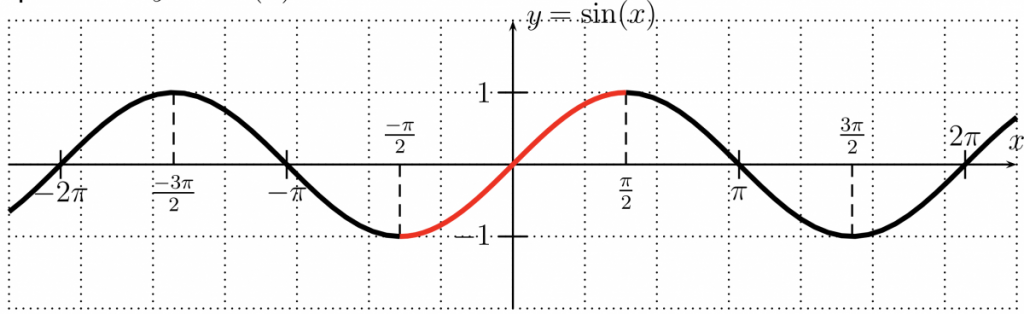
However, if we restrict the function to the interval $\left[-\frac{\pi}{2},\frac{\pi}{2}\right]$ (shown in red) the resulting function is one-to-one, and so we can consider the inverse function.

Definition 19.5. The inverse of the function $y=\sin (x)$ with restricted domain $D=\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]$ and range $R=[-1,1]$ is called the inverse sine or arcsine function. It is denoted by
$y=\sin ^{-1}(x) \quad \text { or } \quad y=\arcsin (x) \quad \Longleftrightarrow \quad \sin (y)=x, \quad y \in\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]$
The arcsine reverses the input and output of the sine function, so that the arcsine has domain $D=[-1,1]$ and range $R=\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]$.

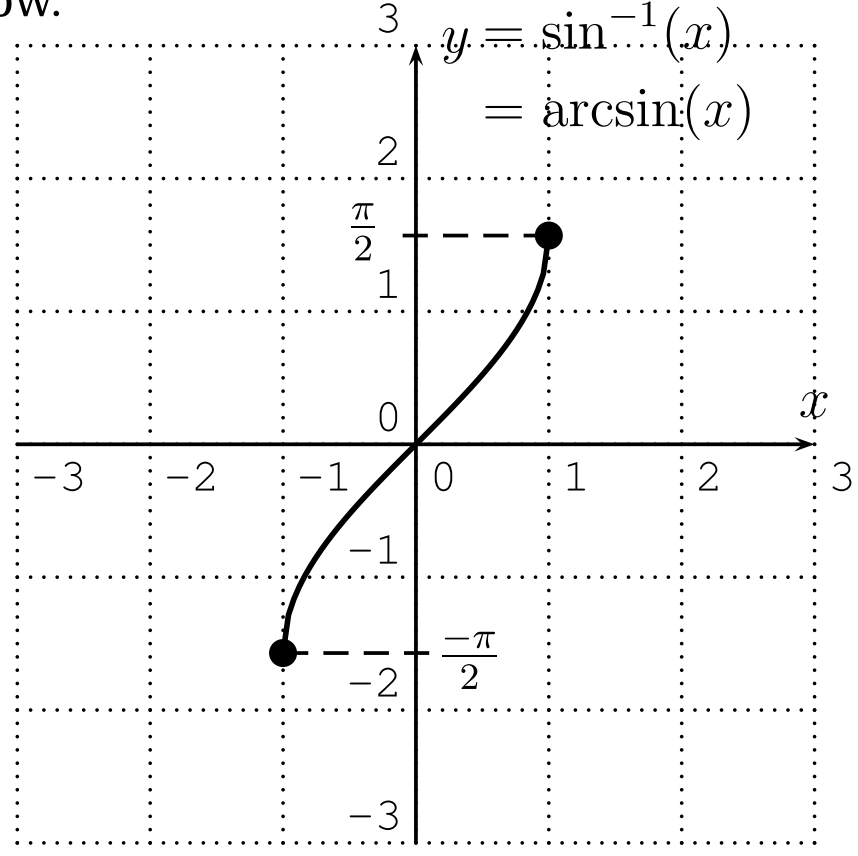
Observation: The inverse sine function is an odd function, so $\sin^{-1}(-x)=-\sin^{-1}(x)$.

Example 19.7. Recall the values of the sine function for common angles:

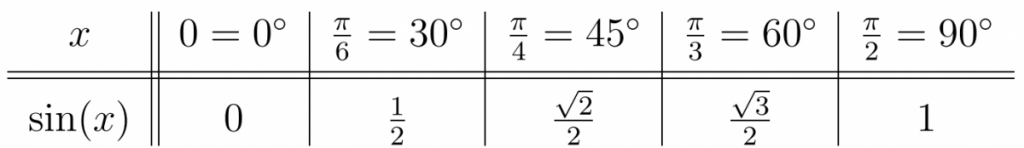
Use the Table and Observation above to find exact values of the arcsine function. Give answers in both degrees and radians.
a. $\sin ^{-1}\left(\frac{\sqrt{2}}{2}\right)$, b. $\sin^{-1}(1)$, c. $\sin^{-1}(0)$, d. $\sin ^{-1}\left(\frac{-1}{2}\right)$, e. $\sin^{-1}(3)$

THE FUNCTION $\cos^{-1}(x)$

We treat the function $\cos(x)$ similar to $\sin(x)$. However, we are no longer able to use the interval $\left[\frac{-\pi}{2}, \frac{\pi}{2}\right]$. Why?

In order to make the cosine function one-to-one, we restrict to the interval $[0,\pi]$.

Definition 19.8. The inverse of the function $y=\cos (x)$ with restricted domain $D=[0, \pi]$ and range $R=[-1,1]$ is called the inverse cosine or arccosine function. It is denoted by
$y=\cos ^{-1}(x) \quad \text { or } \quad y=\arccos (x) \quad \Longleftrightarrow \quad \cos (y)=x, \quad y \in[0, \pi]$
The arccosine reverses the input and output of the cosine function, so that the arccosine has domain $D=[-1,1]$ and range $R=[0, \pi]$.

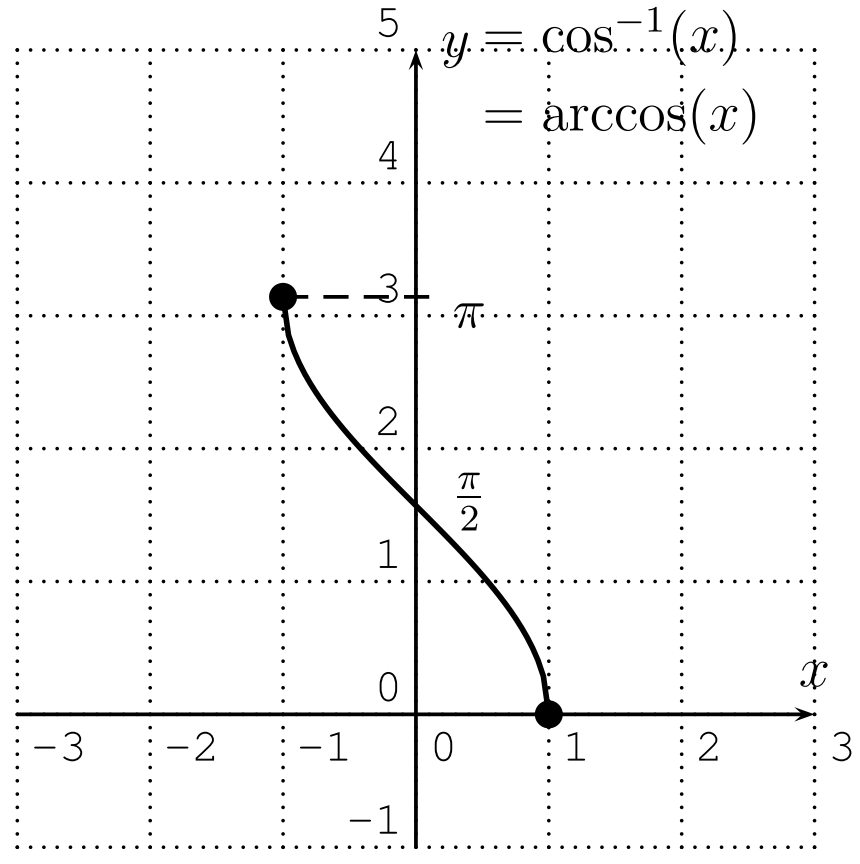
Observation: The arccosine function is neither even nor odd. However, it does obey the following symmetry: $\cos^{-1}(-x)=\pi-\cos^{-1}(x)$
(in many problems, you can avoid the use of this formula by remembering the unit circle definition of cosine).

Example 19.10. Recall the values of the cosine function for common angles:

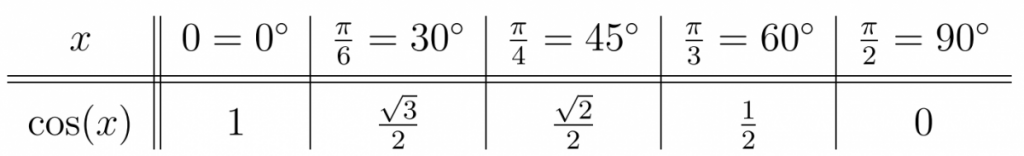
Use the Table and Observation above to find exact values of the arccosine function. Give answers in both degrees and radians.
a. $\arccos\left(\frac{\sqrt{3}}{2}\right)$, b. $\cos^{-1}(1)$, c. $\cos ^{-1}(0)$, d. $\arccos=\left(-\frac{1}{2}\right)$, e. $\arccos(2)$

Inverse trig functions on the TI-84+ calculator

How do we find values of inverse trig functions that don’t appear in our “common angles” table?

Example. Find the values of the inverse trig functions using a calculator. Include at least 5 decimal digits past the decimal point.

a. $\arccos(0.35)$ (in radians)
b. $\tan^{-1}(-13.2)$ (in degrees)