Category: Resources (Page 5 of 10)

Today’s first quiz problem started with an indefinite integral along with specific choices for $u$ and $dv$. With $u=t$, then $du$ is the derivative: $du=1dt$. With $dv=\cos (5t)dt$, then $v$ is antiderivative: $v=\int \cos (5t)dt$.

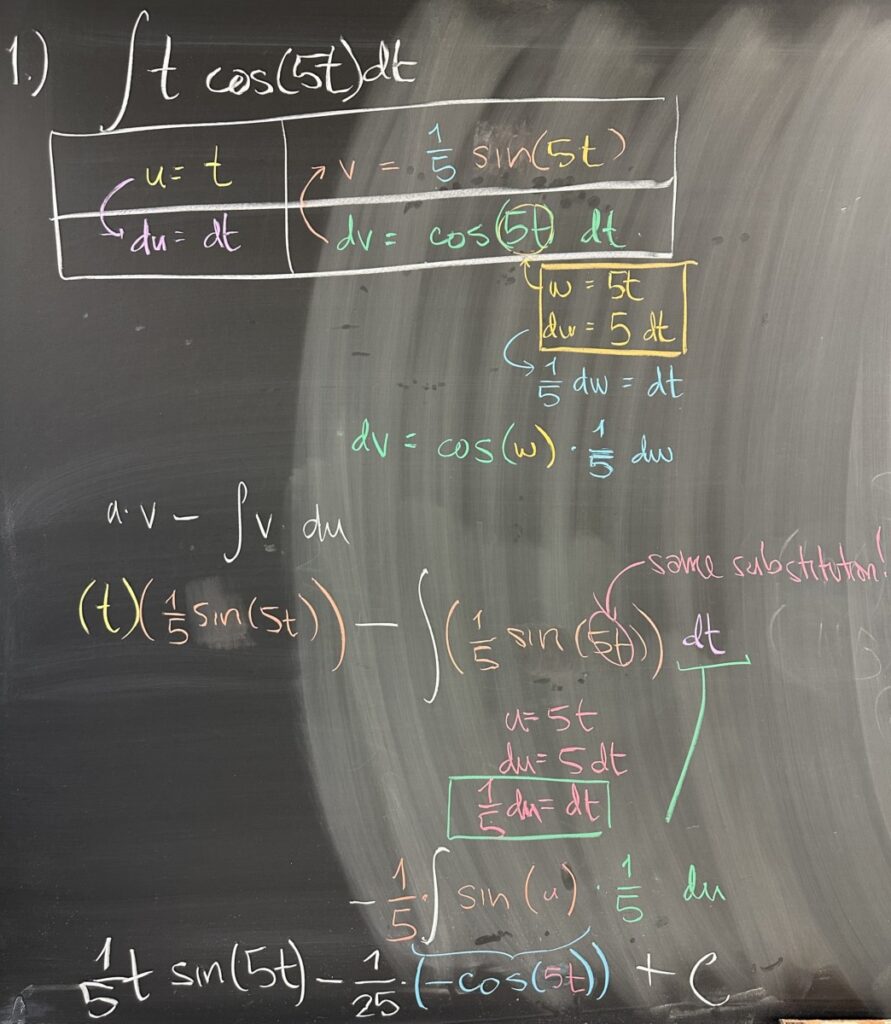
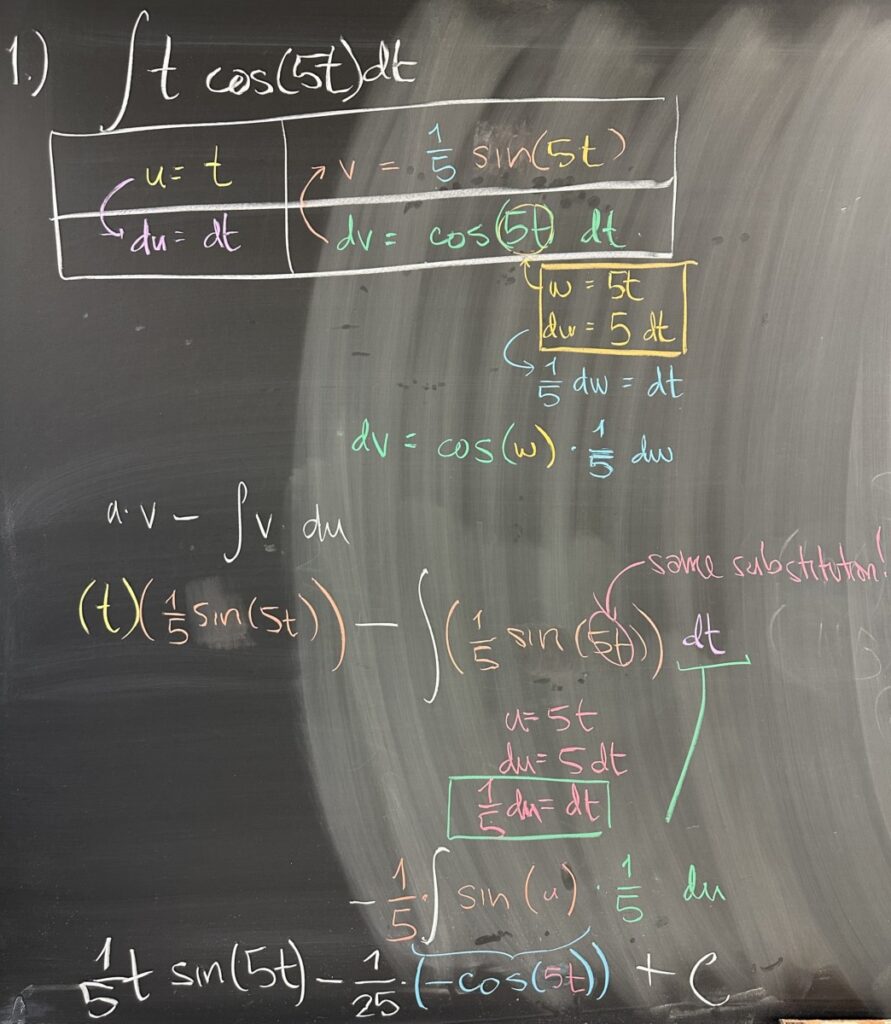
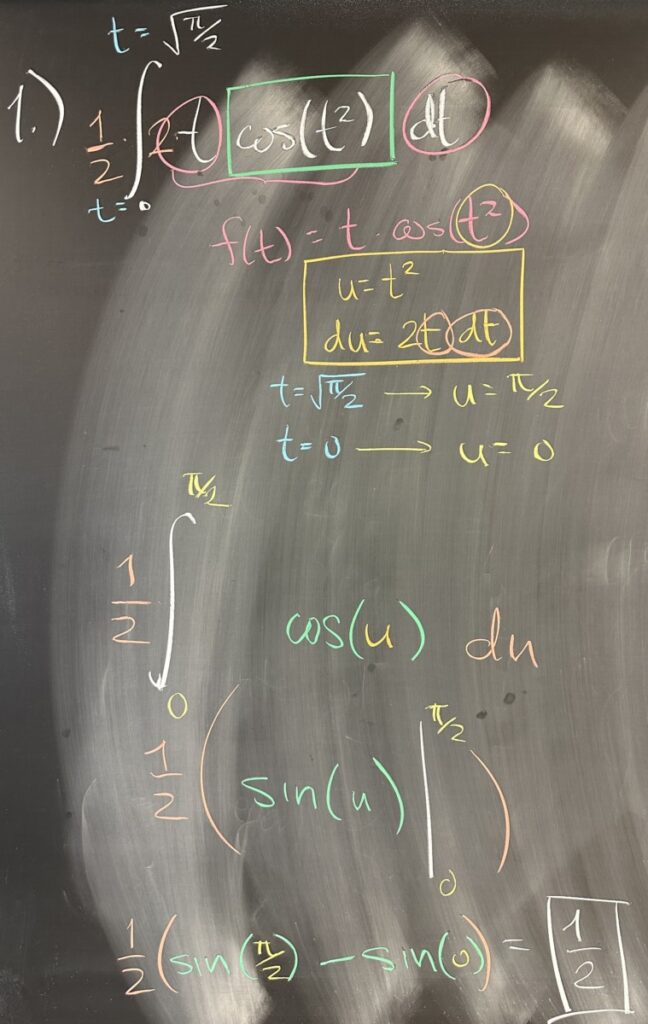
Today’s first quiz problem is a definite integral. It is important to consider what our final result should look like — in this case, as a definite integral, our result should be a real number (representing the area bounded by $f(x)$, the $x$-bounds, and the $x$-axis).

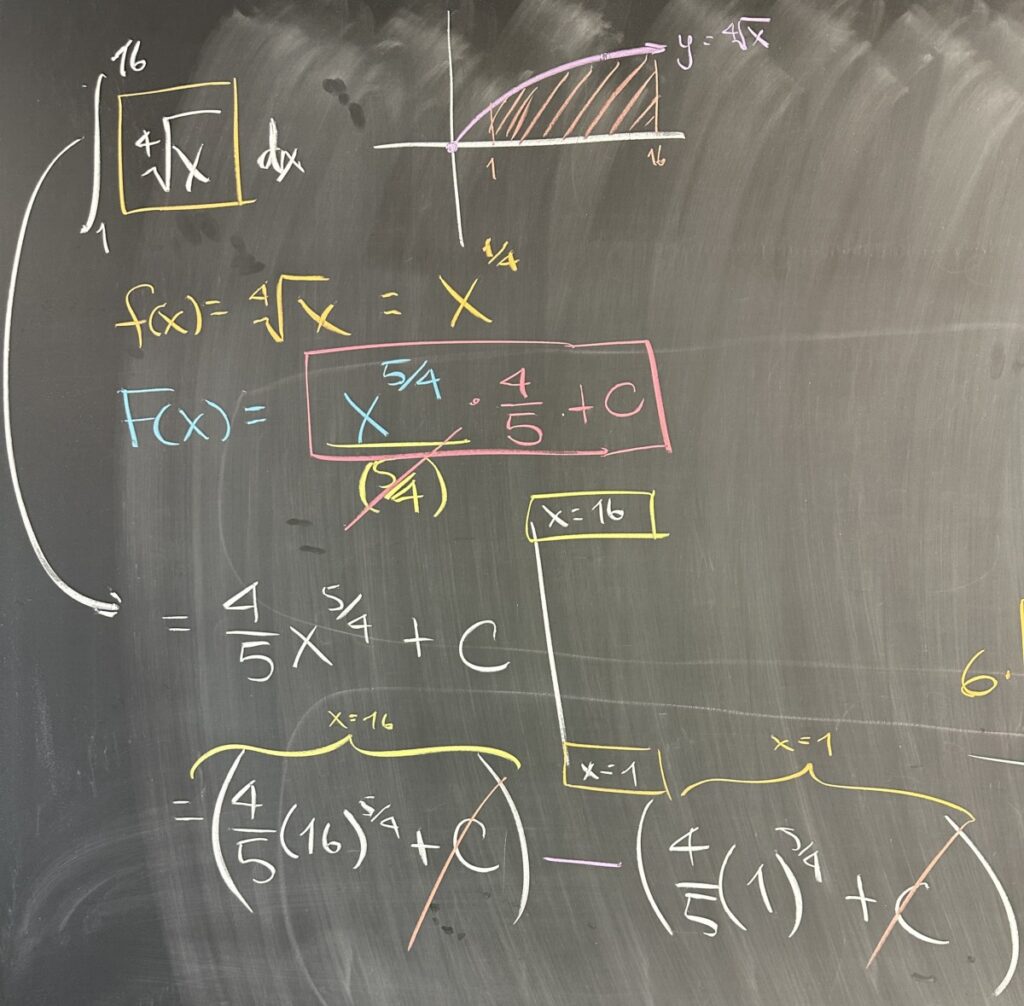
The first quiz problem is a definite integral. We started by thinking about what the function $f(x)=\sqrt[4]{x}$ looks like when graphed, with the bounds $x=1$ and $x=16$. This region is not a “nice” geometric shape — so computing the area will require the Fundamental Theorem of Calculus.