Month: March 2017 (Page 1 of 2)

a=superman is able to prevent evil

b= superman is impotent

c=superman is willing to prevent evil

d=superman is malevolent

e= superman exsists

f= superman prevents evil

argument form=

(a∧c)→f

¬a→b

¬c→d

¬f

e→¬b∧¬d

___________________

:.¬e

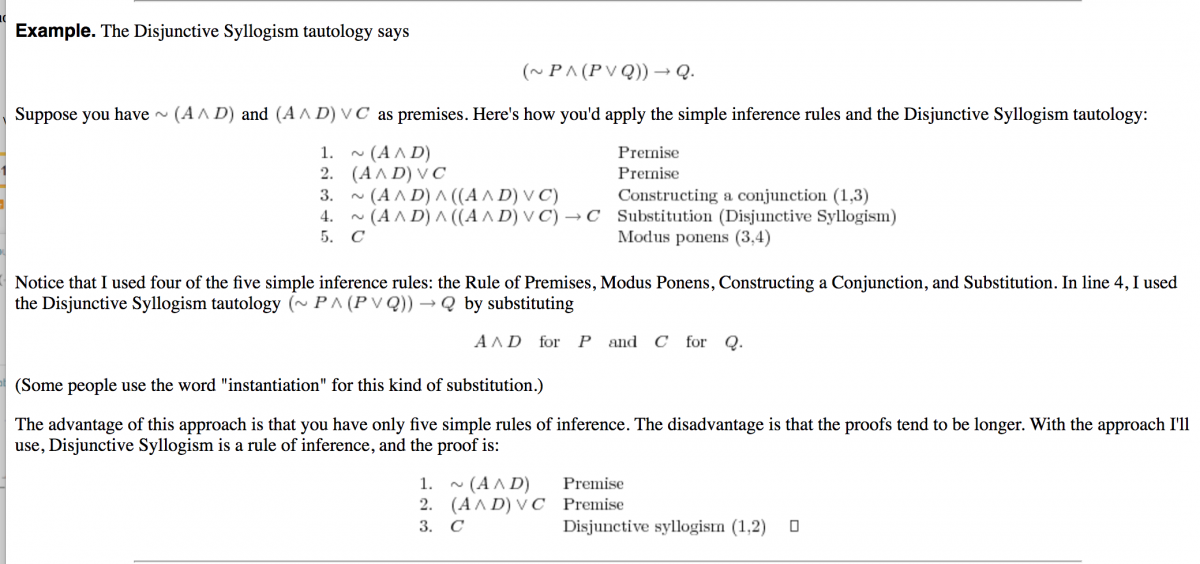
Argument =

(we have to prove ¬e)

1.  (a∧c)→f    =”p”→f  =add  !f  =  apply modus tollens   (!f∧(p→f))→!p = !p = !(a∧c)
2.  ¬ (a∧c)  =  !a V !c

¬a→b

¬c→d

e→¬(b∧d)

*i am lost after this

i know this is supposed to look like this

and pg 73 of the book has it but i dont really understand how the substituting works

this website explains more about building arguments:

http://sites.millersville.edu/bikenaga/math-proof/rules-of-inference/rules-of-inference.html

3a

T(a,b) = Student a likes TV show b where the domain for a is the students in the class and the domain for b is the shows currently airing on TV

Translate:

∀s∃t∃u(T(s,u)→¬T(t,u))

T(s,u) = all students s like a TV show u

or everybody likes a TV show

¬T(t,u) =  it is not the case that a student t likes the TV show u

its not true that one student likes the TV show

(T(s,u)→¬T(t,u))

if everybody likes a TV show, then its not true that one student likes the TV show,

* can this be translated into :

if everybody likes a TV show, then there is not one student who doesnt like the TV show?

3b

use quantifiers and variable to translate the following prop

there is a TV show that everybody likes.

on the test i put this:

∀x∃y (T(x,y))

*should it be

∃y∀x (T(x,y))?

4a. Prove that if x is a rational number and x ≠ 0 then 1/x is a rational number

If x is a rational number ≠ 0, then it can be written as a/b, where a and b are integers and ≠ 0. Then 1/x is equal to 1/(a/b) which equals b/a. Therefore if x is a rational number, 1/x is also rational.

4b. Prove that if x is an irrational number and x≠ 0 then 1/x is an irrational number.

Assume that x is irrational and 1/x is rational. Then 1/x can be written as a/b where a and b are integers ≠ 0. So x is equal to 1/(a/b) which equals b/a, b and a are integers. Therefore x is rational. This contradicts the assumption that x is irrational. Therefore it is true that if x is irrational, 1/x is also irrational.

6a. Determine the argument form of the above argument.

Notation: W(x): x is willing to prevent evil

A(x): x is able to prevent evil

P(x): x prevents evil

I(x): x is impotent

M(x): x is malevolent

E(x): x exists.

S stands for Superman.

Let’s write the givens:

1: (A(S) ∧ W(S)) → P(S)

2. ¬A(S) → I(S)

3. ¬W(S) → M(S)

4. ¬P(S)

5. E(S) → (¬M(S) ∧ ¬I(S))

6. ¬A(S) ∨ ¬W(S)

7. M(S) ∨ I(S)

8. ¬(¬M(S) ∧ ¬I(S))

9. ¬E(S)

To share your solution to a question from test #1 on the OpenLab, add a new post with the question and your full solution (you can upload a photo of your hand-written work if you don’t want to type out the solution). Don’t forget to select the category “Test #1 Solutions” from the right-hand side of the screen.

You may post a solution to a question that has already been solved in someone else’s post, as long as your method for solving is different.

Please comment on others’ posts if you see something that is not correct, unclear, or could otherwise be improved.

The point of this exercise is to get practice writing clear and complete solutions and to get feedback on your attempts. You will get participation credit for your posts and thoughtful comments.