Category: Test #2 Solutions (Page 1 of 6)

https://drive.google.com/file/d/1nygC0NkRdd1wwuBdjWaiEE5B0dEWrua0/view?usp=sharing

Image is too big so I had to share via Google Drive

Storytime:

First you take the Characteristic Equation: r^2 -7r -10 =0. You then factor it and get the roots ( r1=5 & r2=2). Then you follow case 1 and you get the answer from part A (shown above). For part B you take the derivative of part A, you then plug in the initial values given and solve for C1 and C2. Then you plug it back into Yc and that’s your answer.

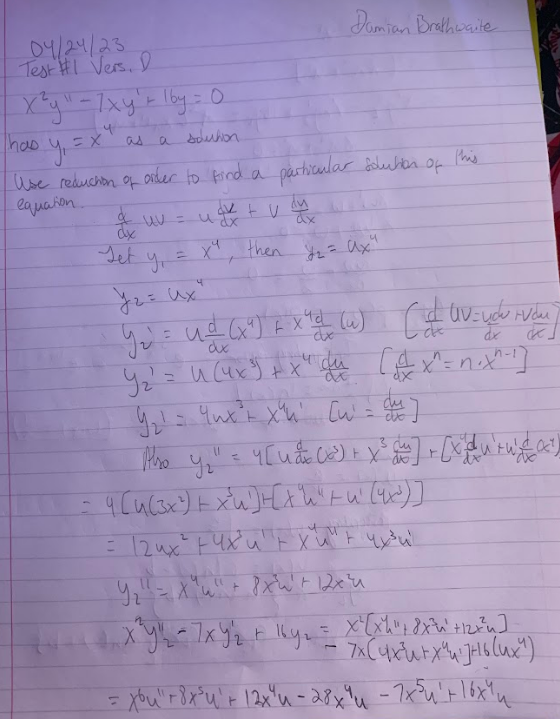
The first step to solving this equation was to use integration by parts, I let y1 = x^4 and y2 = ux^4. Then I would find the first and second derivatives of y2. After finding those I would plug it back into the equation and simplify. While doing this I would also need to use integration by parts again. Next using integrating factors to further get closer to the solution I will find that w(x) = a/x, and then I will integrate that to get the final solution that I would then plug back into the homogeneous general solution.